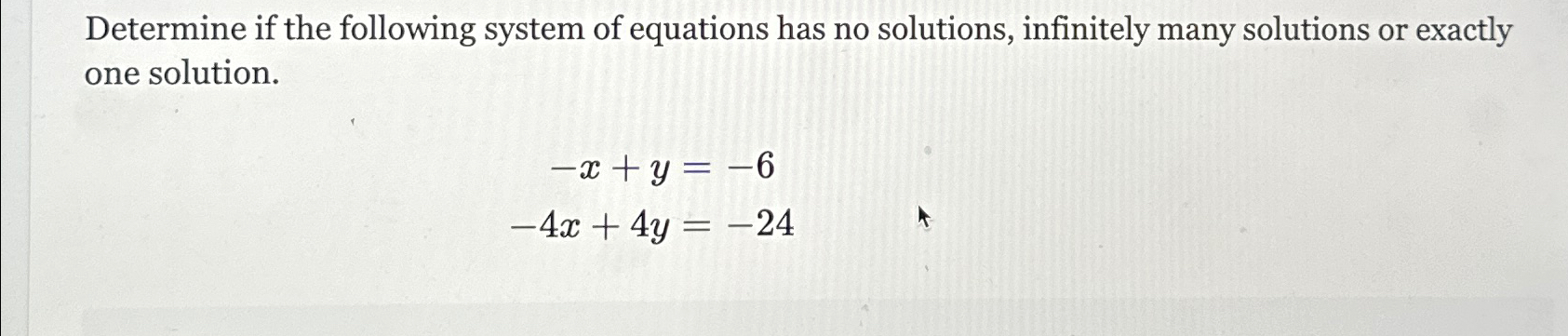
Solved Determine If The Following System Of Equations Has No Chegg To determine if the given system of equations has no solutions, infinitely many solutions, or exactly one solution, multiply the first equation by 10 and the second equation by 5 in order to compare them more easily. Determine if the following system of equations has no solutions, infinitely many solutions or exactly one solution. 4x 3y=7 12x 10y= 27 your solution’s ready to go! our expert help has broken down your problem into an easy to learn solution you can count on.

Solved Problem 1 Consider The Following System Of Chegg To determine if the system has no solutions, infinitely many solutions, or exactly one solution, we can add the two equations together. this simplifies to: the result 0 = 0 is a true statement, which means the system of equations is dependent. Determine if the following system of equations has no solutions, infinitely many solutions or exactly one solution. x 6y=7 6x 30y= 34 there are 2 steps to solve this one. who are the experts?. To solve a system of equations by elimination, write the system of equations in standard form: ax by = c, and multiply one or both of the equations by a constant so that the coefficients of one of the variables are opposite. then, add or subtract the two equations to eliminate one of the variables. Multiply the first equation by 4 and the second by 3: 12x 12y = 4h. 12x 3ky = 3. add the equations: ( 12 3k)y = 4h 3. no solution if 12 3k = 0 and 4h 3 ≠ 0. k = 4 and h ≠ 3 4.
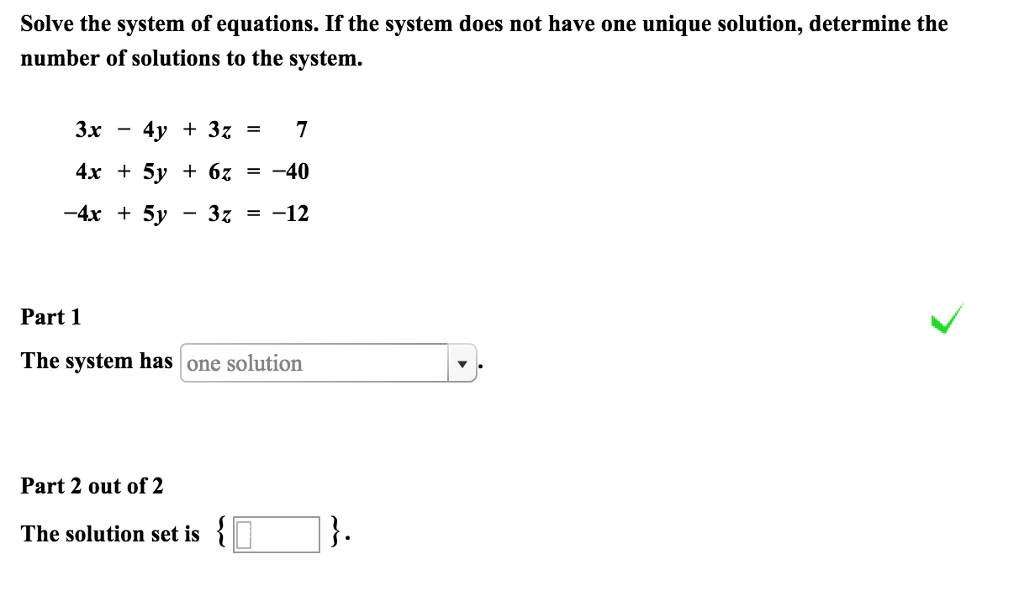
Solved Solve The System Of Equations If The System Does Not Chegg To solve a system of equations by elimination, write the system of equations in standard form: ax by = c, and multiply one or both of the equations by a constant so that the coefficients of one of the variables are opposite. then, add or subtract the two equations to eliminate one of the variables. Multiply the first equation by 4 and the second by 3: 12x 12y = 4h. 12x 3ky = 3. add the equations: ( 12 3k)y = 4h 3. no solution if 12 3k = 0 and 4h 3 ≠ 0. k = 4 and h ≠ 3 4. To determine if the system of equations has no solutions, infinitely many solutions, or exactly one solution, we can use the method of substitution or elimination. let's proceed step by step: we have the system: − x y = 7; x − 4 y = − 9; step 1: solve one equation for one variable. from the first equation, − x y = 7, we solve for y. Learn how to determine if a system of linear equations has no solution or infinitely many solutions. understand the conditions and methods to find the solutions, including solved examples. To determine if a system of equations has no solutions, infinitely many solutions, or exactly one solution, we can use the method of comparison or substitution. in this case, we can see that the second equation is a multiple of the first equation. This calculator solves systems of linear equations with steps shown, using gaussian elimination method, inverse matrix method, or cramer's rule. also you can compute a number of solutions in a system (analyse the compatibility) using rouché–capelli theorem .
