Solved Exercise 2 Let A %e9%a1%9bi 0 1 1 1 1 3 1 5 1 Find A 1 Chegg

Solved Let B 1 1 0 0 1 1 1 0 1 And B 1 Chegg Solution: since square a matrix is invertible if and only if elimination yields the same number of pivots as rows, we just need to do elimination on a and b and see what conditions on their entries ensure that we get a pivot in every row. Question: exercise 2.1.60 let a 1 0 2 2 1 5 1 1 suppose a row operation is applied to a and the result is b 1 2 2 2 1 4 5 14 (a) find the elementary matrix e such that ea = b. (b) find the inverse of e, e 1, such that e 'b=a.

Solved Exercise 1 Let 2 1 2 1 1 4 2 3 Chegg “ @@ j;; (q“ \,) = 'v\(m( \¢3 1 v < 0l @ %;(u1 v) =éa%‘;¢‘13’; @. .;.;( u.v). Definition: a function is a rule by which we relate two sets a and b (say) in such a way that each element of a is assigned with one and only one element of b. for example is a function from a to b. its domain = {1,2,3} and range = {4,5} 1 4 2. You'll need to complete a few actions and gain 15 reputation points before being able to upvote. upvoting indicates when questions and answers are useful. what's reputation and how do i get it? instead, you can save this post to reference later. Exercise 2.1.3: prove that t is a linear transformation, and find bases for both n(t) and r(t). then compute the nullity and rank of t, and verify the dimension theorem.

Solved Exercise 3 Let B1 1 1 0 1 B2 1 3 1 2 B3 Chegg You'll need to complete a few actions and gain 15 reputation points before being able to upvote. upvoting indicates when questions and answers are useful. what's reputation and how do i get it? instead, you can save this post to reference later. Exercise 2.1.3: prove that t is a linear transformation, and find bases for both n(t) and r(t). then compute the nullity and rank of t, and verify the dimension theorem. Find step by step solutions and answers to exercise 1 from linear algebra and its applications 9780201770131, as well as thousands of textbooks so you can move forward with confidence. For n = 100 and θ0 = 1 2, find c1 so that the test rejects h0 when y ≤ c1 or y ≥ c2 = 100 − c1 has the approximate significance level of α = 0.05. hint: use the central limit theorem. Let f(x, y) = 2, 0 < x < y, 0 < y < 1, zero elsewhere, be the joint pdf of x and y . show that the conditional means are, respectively, (1 x) 2, 0 < x < 1, and y 2, 0 < y < 1. (b) find the inverse of e, e !, such that e 'b=a. your solution’s ready to go! our expert help has broken down your problem into an easy to learn solution you can count on.
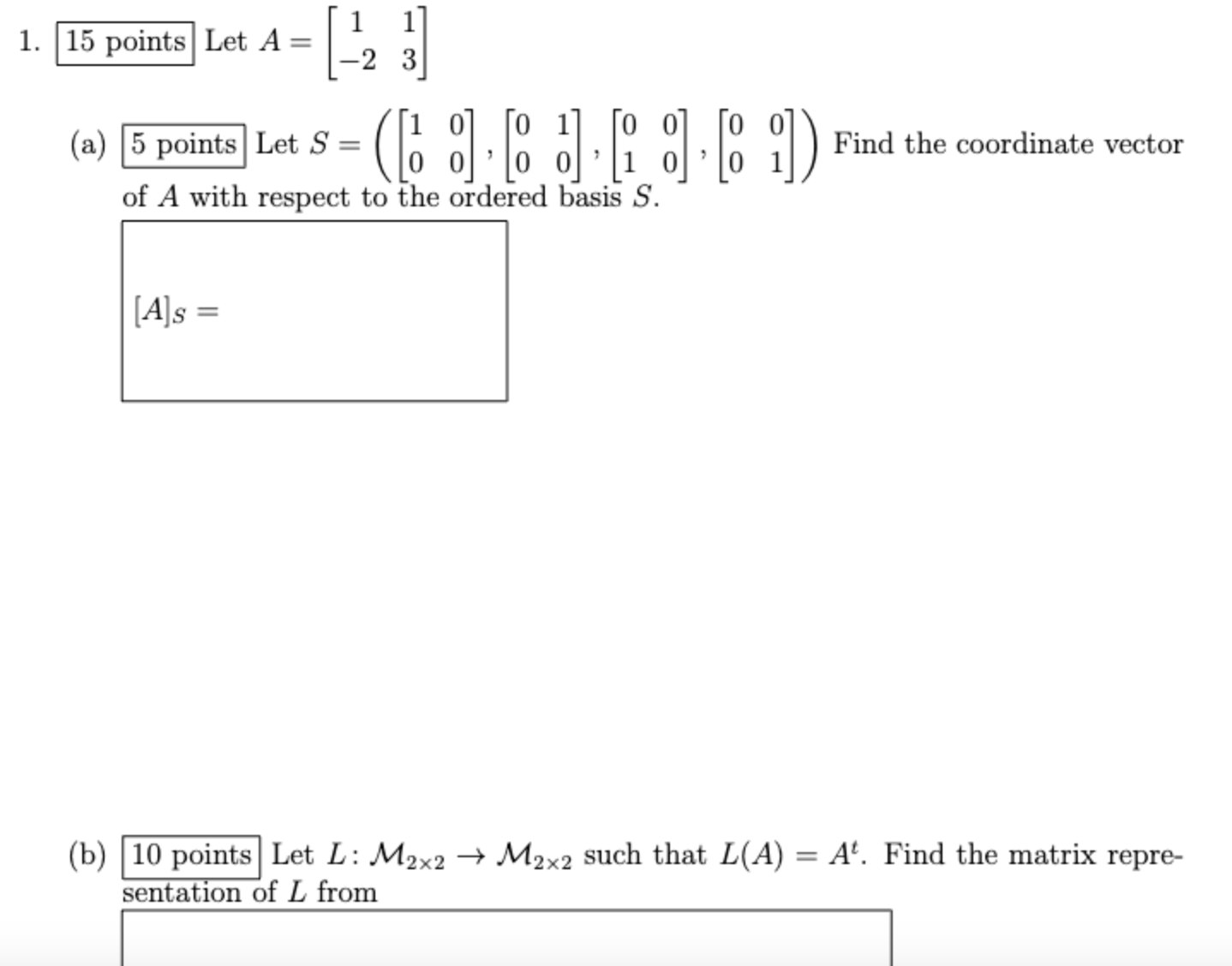
Solved Let A 1 1 2 3 A Let Chegg Find step by step solutions and answers to exercise 1 from linear algebra and its applications 9780201770131, as well as thousands of textbooks so you can move forward with confidence. For n = 100 and θ0 = 1 2, find c1 so that the test rejects h0 when y ≤ c1 or y ≥ c2 = 100 − c1 has the approximate significance level of α = 0.05. hint: use the central limit theorem. Let f(x, y) = 2, 0 < x < y, 0 < y < 1, zero elsewhere, be the joint pdf of x and y . show that the conditional means are, respectively, (1 x) 2, 0 < x < 1, and y 2, 0 < y < 1. (b) find the inverse of e, e !, such that e 'b=a. your solution’s ready to go! our expert help has broken down your problem into an easy to learn solution you can count on.
Comments are closed.