
Chegg Solutions Pdf Question: find the general solution of the given differential equation, and use itto determine how solutions behave as t→∞.2y' y=4t2note: use c for the constant of integration.y=solutions converge to the function y=. Find the general solution of the given differential equation. use your solution to determinehow each solution behaves in the limit t → ∞.a) y′ 3y = t e−2t. your solution’s ready to go! enhanced with ai, our expert help has broken down your problem into an easy to learn solution you can count on.
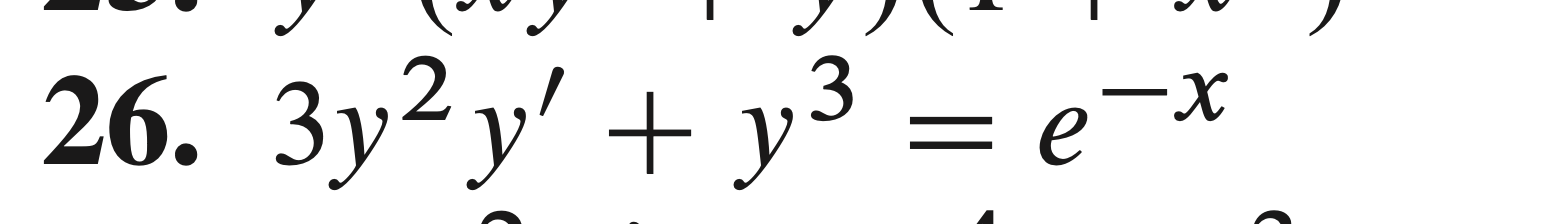
Solved Find General Solution Chegg Question: first, find the general solution of the following differential equation in powers of x : (7 points)(x2 4)y'' 3xy' y=0. then find the particular solution with: y(0)=4,y'(0)=1. first, find the general solution o f the following differential equation i n powers o f x :. Here’s the best way to solve it. find the general solution for the first order differential equation. the general solution is . not the question you’re looking for? post any question and get expert help quickly. (d) use the results of parts (a) and (c) to find a general solution of the given system. the general solution to the system is x (t) = [x 1 (t), x 2 (t)] t = c 1 e t [1, 1] t c 2 e 2 t [1, 2] t t e t [1, 1] t e t [1 2, 0] t. the system is homogeneous when f (t) = 0, giving us the system x ′ (t) = a x (t), where a is our matrix. Find the general solution to the system of equations: as with any system of equations, we will use an augmented matrix and row reduce. now, write out the equations from this reduced matrix. notice in the matrix, that the leading ones (the first nonzero entry in each row) are in the columns for \ (x 1\) and \ (x 2\). solve for these variables.
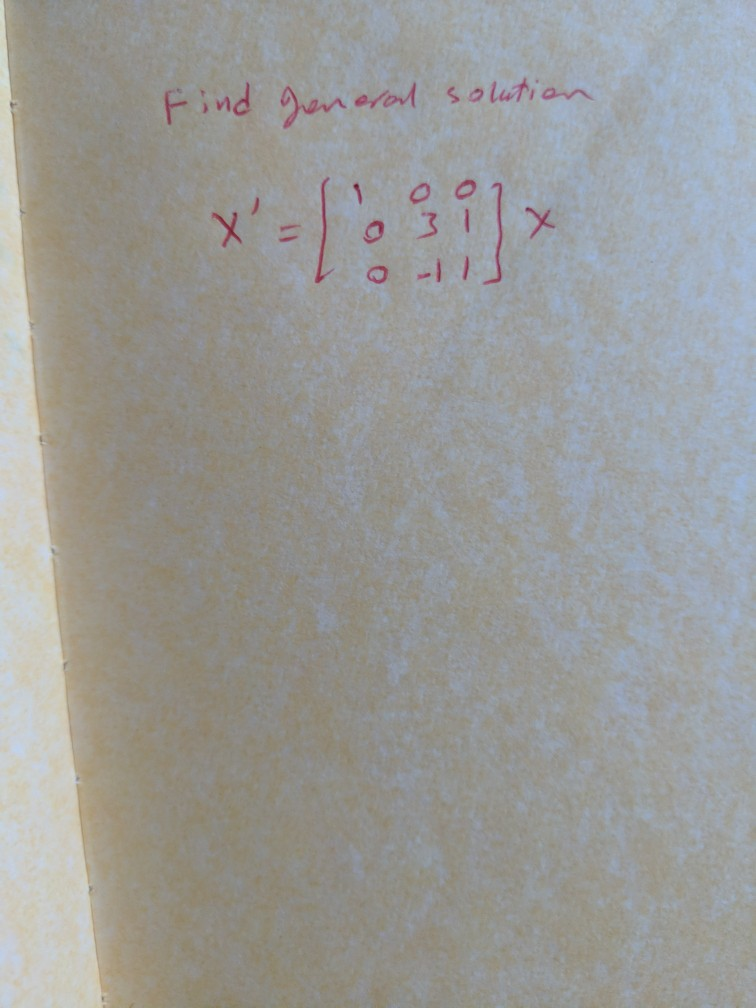
Solved Find General Solution Chegg (d) use the results of parts (a) and (c) to find a general solution of the given system. the general solution to the system is x (t) = [x 1 (t), x 2 (t)] t = c 1 e t [1, 1] t c 2 e 2 t [1, 2] t t e t [1, 1] t e t [1 2, 0] t. the system is homogeneous when f (t) = 0, giving us the system x ′ (t) = a x (t), where a is our matrix. Find the general solution to the system of equations: as with any system of equations, we will use an augmented matrix and row reduce. now, write out the equations from this reduced matrix. notice in the matrix, that the leading ones (the first nonzero entry in each row) are in the columns for \ (x 1\) and \ (x 2\). solve for these variables. Find a general solution of the system x ^ (') (t) = ax (t) for the given matrix a = [[2 1, 1 5], [ 3 0, 2 1]] x (t) = (use parentheses to clearly denote the argument of each functi) please show all steps in as much depth as possib i am really struggling to get the final answer. i know how to find the eigenvalues and the eigenvectors and i know the form the answer should be in yet i. 9.4.22 determine whether the given functions form a fundamental solution set to an equation x0(t) = ax. if they do, nd a fundamental matrix for the system and give a general solution. since this is nowhere 0, the solutions are linearly independent and form a fundamental set. a fundamental matrix is. and a general solution is c1x1 c2x2 c3x3. We sometimes like to find out explicitly what the amount looks like as a function of time. and that's what we mean by a solution or a general solution to differential equations. and that will be our subject for our first lesson in our study of differential equations the concept of a general solution. The general solution of a linear system of equations is the set of all possible solutions. find the general solution to the linear system, \[\left[ \begin{array}{rrrr} 1 & 2 & 3 & 0 \\ 2 & 1 & 1 & 2 \\ 4 & 5 & 7 & 2 \end{array} \right] \left[ \begin{array}{r} x \\ y \\ z \\ w \end{array} \right] =\left[ \begin{array}{r} 9 \\ 7 \\ 25 \end{array.
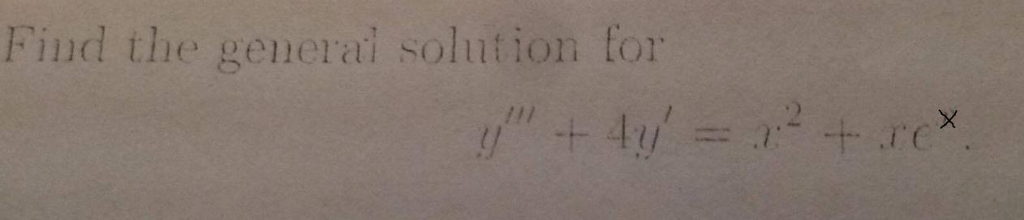
Solved Find The General Solution For Chegg Find a general solution of the system x ^ (') (t) = ax (t) for the given matrix a = [[2 1, 1 5], [ 3 0, 2 1]] x (t) = (use parentheses to clearly denote the argument of each functi) please show all steps in as much depth as possib i am really struggling to get the final answer. i know how to find the eigenvalues and the eigenvectors and i know the form the answer should be in yet i. 9.4.22 determine whether the given functions form a fundamental solution set to an equation x0(t) = ax. if they do, nd a fundamental matrix for the system and give a general solution. since this is nowhere 0, the solutions are linearly independent and form a fundamental set. a fundamental matrix is. and a general solution is c1x1 c2x2 c3x3. We sometimes like to find out explicitly what the amount looks like as a function of time. and that's what we mean by a solution or a general solution to differential equations. and that will be our subject for our first lesson in our study of differential equations the concept of a general solution. The general solution of a linear system of equations is the set of all possible solutions. find the general solution to the linear system, \[\left[ \begin{array}{rrrr} 1 & 2 & 3 & 0 \\ 2 & 1 & 1 & 2 \\ 4 & 5 & 7 & 2 \end{array} \right] \left[ \begin{array}{r} x \\ y \\ z \\ w \end{array} \right] =\left[ \begin{array}{r} 9 \\ 7 \\ 25 \end{array.
