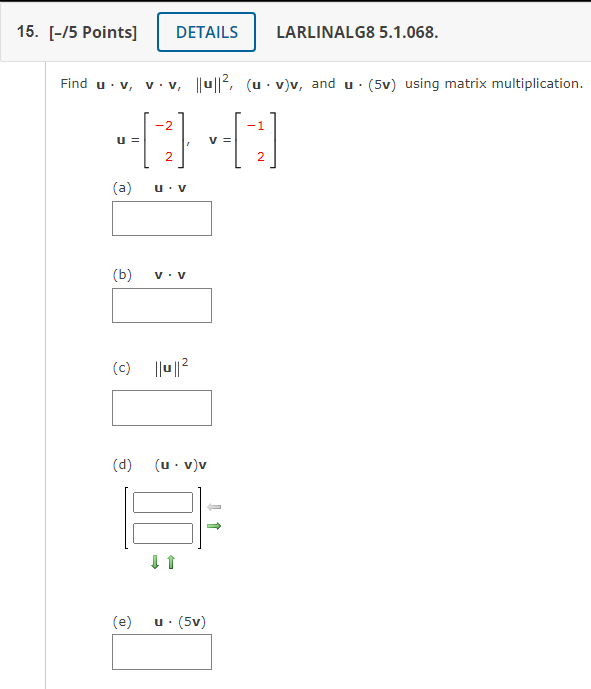
Solved Find U V V V U 2 U V V ï And U 5v ï Using Matrix Chegg Our expert help has broken down your problem into an easy to learn solution you can count on. here’s the best way to solve it. to find u ⋅ v, calculate the sum of the products of the corresponding components of the vectors u and v: (0 ⋅ 4) (2 ⋅ − 3) (4 ⋅ 5) (5 ⋅ 0). not the question you’re looking for?. Find (3u v) . (u iv), given that u . u = 5, u v = 6, and v v = 8. question: find u v, v v, ||u |2, (u v)v, and u (5v). u= (5, 4, 0, 5), v= (4, 3, 2,0) (a) uv (b) vv (c) l2 (d) (u v)v (e) u (5v) question details larlinalg8 5.1.028. find (3u v) . (u iv), given that u . u = 5, u v = 6, and v v = 8. there are 3 steps to solve this one.
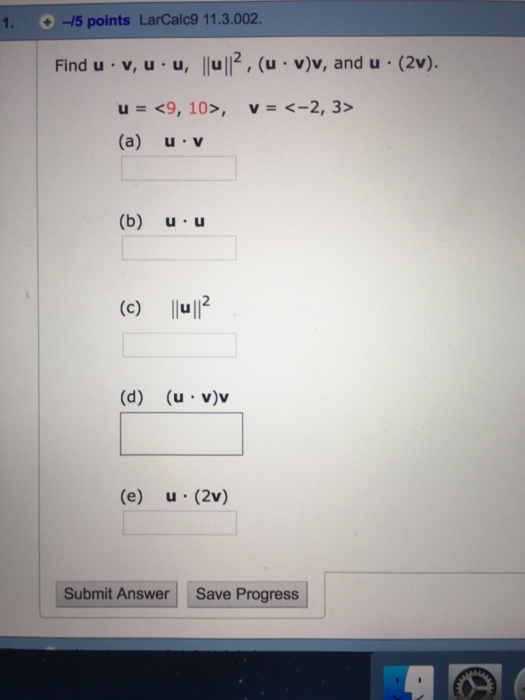
Solved Find U V U U U 2 U V V And U 2v U Chegg U^2 = (3^2, 2^2) = (9, 4). (u · v)v (scalar projection of u onto v): to find the scalar projection of u onto v, we first calculate the dot product of u and v, and then multiply the result by v. (u · v)v = 6 * (4, 3) = (24, 18). u · (5v) (vector projection of 5v onto u): to find the vector projection of 5v onto u, we multiply 5v by the. The product of vector u with 5v, u.(5v), is found by multiplying each component of u by 5 times each component of v. explanation: to find the dot product between two vectors, u and v, we multiply the corresponding components and then sum them up. so, u·v = ( 3)(2) (4)(3) = 6 12 = 6. The dot product of u.v is 6, 12). the dot product of v.v is (4, 16). the dot product of u² is (9, 9). the dot product of (u·v)v is (12, 48). the dot product of u·(5v) is (30, 60). what is the dot product of the vector? the dot product of the vectors is calculated as follows; the given vectors; u = (3, 3) v = (2, 4). Calculate the dot product \( u \cdot v \) using the formula for the dot product of two vectors. calculate the dot product \( v \cdot v \) using the same formula. compute the scalar multiplication of vector \( u \) by \( \frac{1}{2} \).

Solved Find U V V V U 2 U V V ï And Chegg The dot product of u.v is 6, 12). the dot product of v.v is (4, 16). the dot product of u² is (9, 9). the dot product of (u·v)v is (12, 48). the dot product of u·(5v) is (30, 60). what is the dot product of the vector? the dot product of the vectors is calculated as follows; the given vectors; u = (3, 3) v = (2, 4). Calculate the dot product \( u \cdot v \) using the formula for the dot product of two vectors. calculate the dot product \( v \cdot v \) using the same formula. compute the scalar multiplication of vector \( u \) by \( \frac{1}{2} \). There are 3 steps to solve this one. calculate the dot product of the given vectors u and v, denoted as u.v, by multiplying the corresponding components of the vectors and adding them up. find u v, v v, ||u|2, (u v)v, and u (5v) u (4, 3), v ( 3, 2) (a) u v (b) v v (c) l2 (d) (u v)v (e) u (5v). Find two unit vectors each of which is perpendicular to both u ¯ and v ¯ where u ¯ = 2 i ^ j ^ 2 k ^, v ¯ = i ^ 2 j ^ 2 k ^. if a → and b → are two vectors perpendicular to each other, prove that (a → b →) 2 = (a → b →) 2. To find u.v, we can use the formula for the dot product: u.v = u1v1 u2v2 u3v3 u4v4. substituting the given values: u.v = ( 3) (2) (5) (4) (5) (0) (0) (4) simplifying: u.v = 6 20 0 0 = 14. therefore, u.v = 14. to find v.v, we can use the same formula: v.v = v1v1 v2v2 v3v3 v4v4. substituting the given values:. Find a unit vector in the direction of u and in the direction opposite that of u. we have an expert written solution to this problem! c (3, 4, 2) = 1? (u · v)v, and u · (5v). verify the cauchy schwarz inequality for the vectors. calculate the following values. we draw the following conclusion.

Solved Find U V V V U 2 U V V And U 5v U 4 Chegg There are 3 steps to solve this one. calculate the dot product of the given vectors u and v, denoted as u.v, by multiplying the corresponding components of the vectors and adding them up. find u v, v v, ||u|2, (u v)v, and u (5v) u (4, 3), v ( 3, 2) (a) u v (b) v v (c) l2 (d) (u v)v (e) u (5v). Find two unit vectors each of which is perpendicular to both u ¯ and v ¯ where u ¯ = 2 i ^ j ^ 2 k ^, v ¯ = i ^ 2 j ^ 2 k ^. if a → and b → are two vectors perpendicular to each other, prove that (a → b →) 2 = (a → b →) 2. To find u.v, we can use the formula for the dot product: u.v = u1v1 u2v2 u3v3 u4v4. substituting the given values: u.v = ( 3) (2) (5) (4) (5) (0) (0) (4) simplifying: u.v = 6 20 0 0 = 14. therefore, u.v = 14. to find v.v, we can use the same formula: v.v = v1v1 v2v2 v3v3 v4v4. substituting the given values:. Find a unit vector in the direction of u and in the direction opposite that of u. we have an expert written solution to this problem! c (3, 4, 2) = 1? (u · v)v, and u · (5v). verify the cauchy schwarz inequality for the vectors. calculate the following values. we draw the following conclusion.
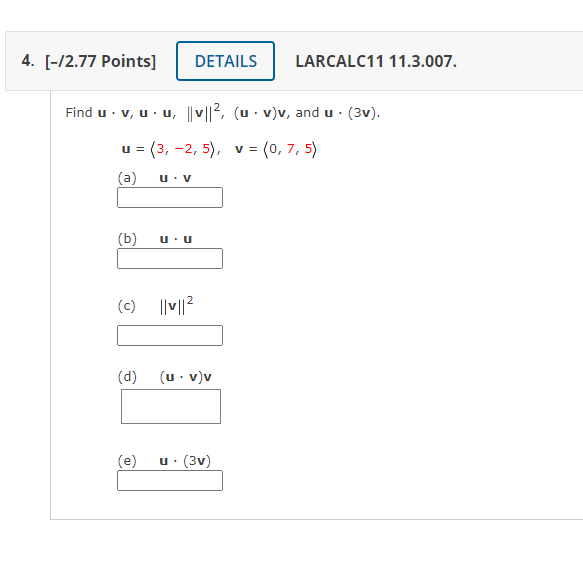
Solved Find U V U U V 2 U Chegg To find u.v, we can use the formula for the dot product: u.v = u1v1 u2v2 u3v3 u4v4. substituting the given values: u.v = ( 3) (2) (5) (4) (5) (0) (0) (4) simplifying: u.v = 6 20 0 0 = 14. therefore, u.v = 14. to find v.v, we can use the same formula: v.v = v1v1 v2v2 v3v3 v4v4. substituting the given values:. Find a unit vector in the direction of u and in the direction opposite that of u. we have an expert written solution to this problem! c (3, 4, 2) = 1? (u · v)v, and u · (5v). verify the cauchy schwarz inequality for the vectors. calculate the following values. we draw the following conclusion.
