
Solved Determine If The Following Sets Of Vectors Are Chegg For each of the following sets of vectors, determine whether or not the set is orthogonal. if the set is orthogonal, normalize it to obtain an orthonormal set if the set is not orthogonal, show this by giving the dot product of an appropriate pair of the vectors. 1. determine whether the given set is a vector space. if not, give at least one axiom that is not satisfied. unless otherwise stated, assume that vector addition and scalar multiplication are the ordinary operations defined on the set. (a) the set of vectors f(a;b) 2r2: b= 3a 1g answer: this is not a vector space. it does not contain the zero.
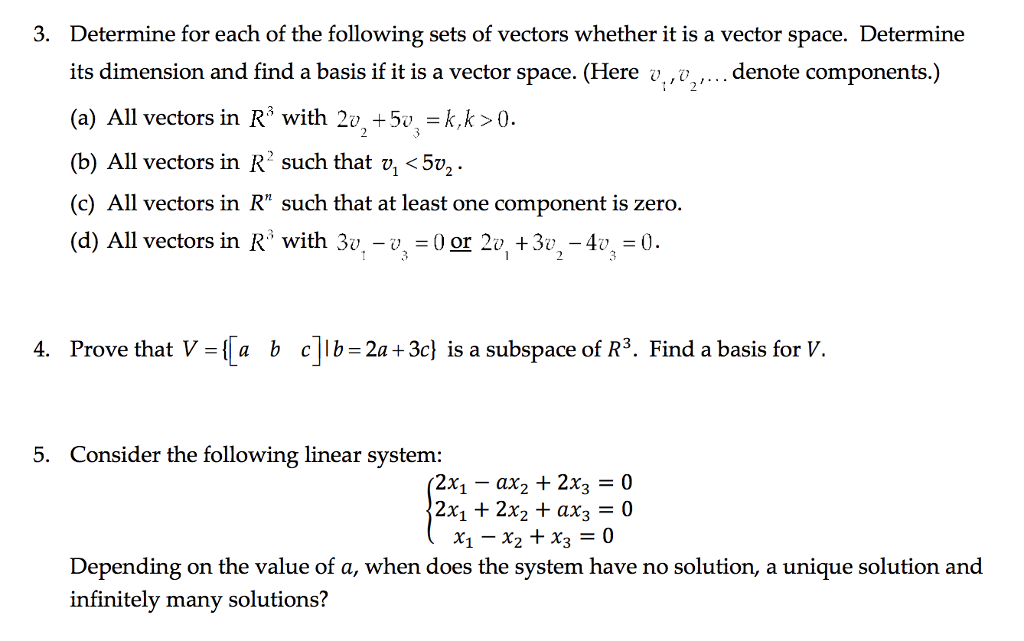
Solved 3 Determine For Each Of The Following Sets Of Chegg • determine whether a set of vectors is linearly independent or linearly dependent. • express one vector in a linearly dependent set as a linear combination of the other vectors in the set. • use the wronskian to show that a set of functions is linearly independent. Determine whether or not each of the following is a subspace of r 2. justify your answer. solution. [4 points] yes, x1 is a subspace. given any (x; y); (x0; y0) 2 x1 and c 2 r, we must check that (cx x0; cy y0) 2 x1. indeed, (cx x0) (cy y0) = c(x y) (x0 y0) = c 0 0 = 0: solution. [4 points] no, x2 is not a subspace. For each of the following sets of vectors, determine whether or not the set is linearly independent. for those that are dependent, write one of the vectors as a linear combination of the others. There are 3 steps to solve this one. let's analyze each set of vectors to determine whether they span r 3 and whether they are linearly inde not the question you’re looking for? post any question and get expert help quickly.

Solved 3 Consider The Following Sets Of Vectors Determine Chegg For each of the following sets of vectors, determine whether or not the set is linearly independent. for those that are dependent, write one of the vectors as a linear combination of the others. There are 3 steps to solve this one. let's analyze each set of vectors to determine whether they span r 3 and whether they are linearly inde not the question you’re looking for? post any question and get expert help quickly. To determine whether the given sets of vectors are linearly independent or dependent, we analyze each case: a) the vectors given are: u = − 1, v = 2, w = 2. to check linear independence, we want to see if there exist scalars a, b, c such that: a (− 1) b (2) c (2) = 0 this simplifies to: − a 2 b 2 c = 0 when we consider the scalars. For each vector in the set, nd whether it lies in the set spanned by the other vectors. answers: 1. linearly independent; no vector is in the span of the other vector. 2. linearly dependent, with a relation 2~a1 ~a2 = 0. therefore, ~a1 = ~a2=2 2 span(~a2) and ~a2 = 2~a1 2 span(~a1). 3. linearly dependent, with a relation ~a1 ~a2 ~a3 = 0. First, let's define the given sets of vectors: a) set a: $\textbf {u} = \begin {bmatrix} 1 \\ 0 \\ 0 \end {bmatrix}$, $\textbf {v} = \begin {bmatrix} 0 \\ 1 \\ 0 \end {bmatrix}$, $\textbf {w} = \begin {bmatrix} 0 \\ 0 \\ 1 \end {bmatrix}$ b) set b: $\textbf {u} = \begin {bmatrix} 1 \\ 1 \\ 0 \end {bmatrix}$, $\textbf {v} = \begin {bmatrix} 0 \. Set of two vectors is linearly independent if and only if neither of the vectors is a multiple of the other. special cases: 3. a set of vectors s = fv1; v2; : : : ; vpg in rn containing the zero vector is linearly dependent.
