
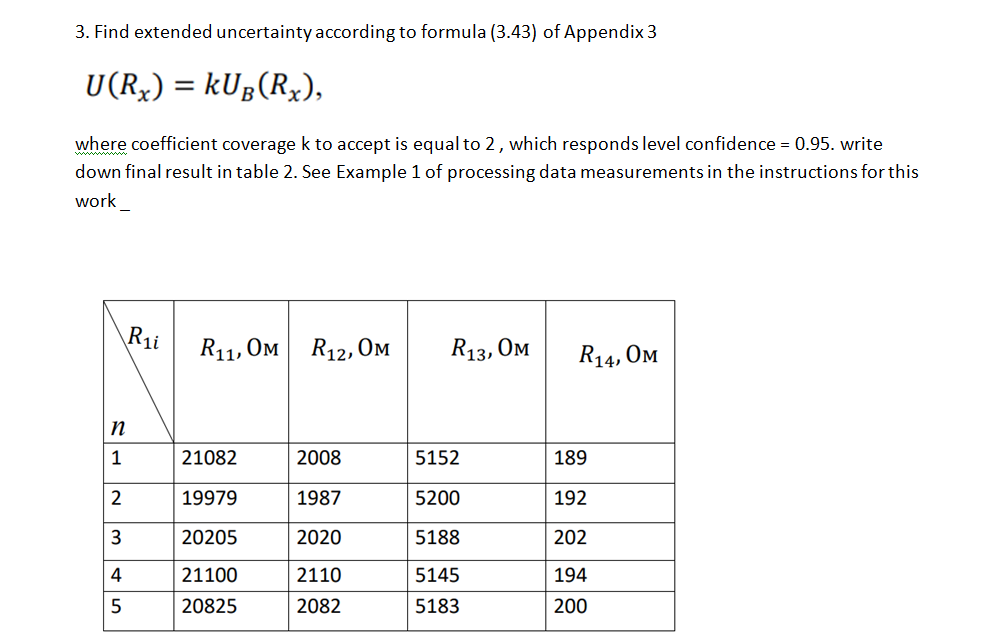
Solved Series Solution Chegg Our expert help has broken down your problem into an easy to learn solution you can count on. solve for the fourier series. there’s just one step to solve this. consider the given function . here the function f has period 2 . not the question you’re looking for? post any question and get expert help quickly. A fourier series for a function f (x) o n the interval l ≤ x ≤ l has the f ( x ) = a 0 2 ∑ n = 1 ∞ ( a n c o s ( n π x l ) b n s i n ( n π x l ) ) for each o f the following problems, calculate the fourier series for the given function f ( x ) and.
Solved Chegg Learn from step by step solutions for over 34,000 isbns in math, science, engineering, business and more. answers in a pinch from experts and subject enthusiasts all semester long. fourier series textbook solutions from chegg, view all supported editions. The solution expresses the fourier series as a sum involving cosine and sine terms. it then sketches the partial sums of the fourier series up to the term including n=3. the document provides a step by step solution to problem 17p in chapter 11.1 of advanced engineering mathematics. Determine the fourier cosine series of the even triangle function represented by fig. \(\pageindex{1}\). solution. view tutorial on . the triangle function depicted in fig. \(\pageindex{1}\) is an even function of \(x\) with period \(2\pi\) (i.e., \(l =\pi\)). its definition on \(0 < x <\pi\) is given by \[f(x)=1 \frac{2x}{\pi}.\nonumber\]. 0 2 in the fourier series. this allows us to represent functions that are, for example, entirely above the x−axis. with a sufficient number of harmonics included, our ap proximate series can exactly represent a given function f(x) f(x) = a 0 2 a 1 cosx a 2 cos2x a 3 cos3x b 1 sinx b 2 sin2x b 3 sin3x toc jj ii j i back.

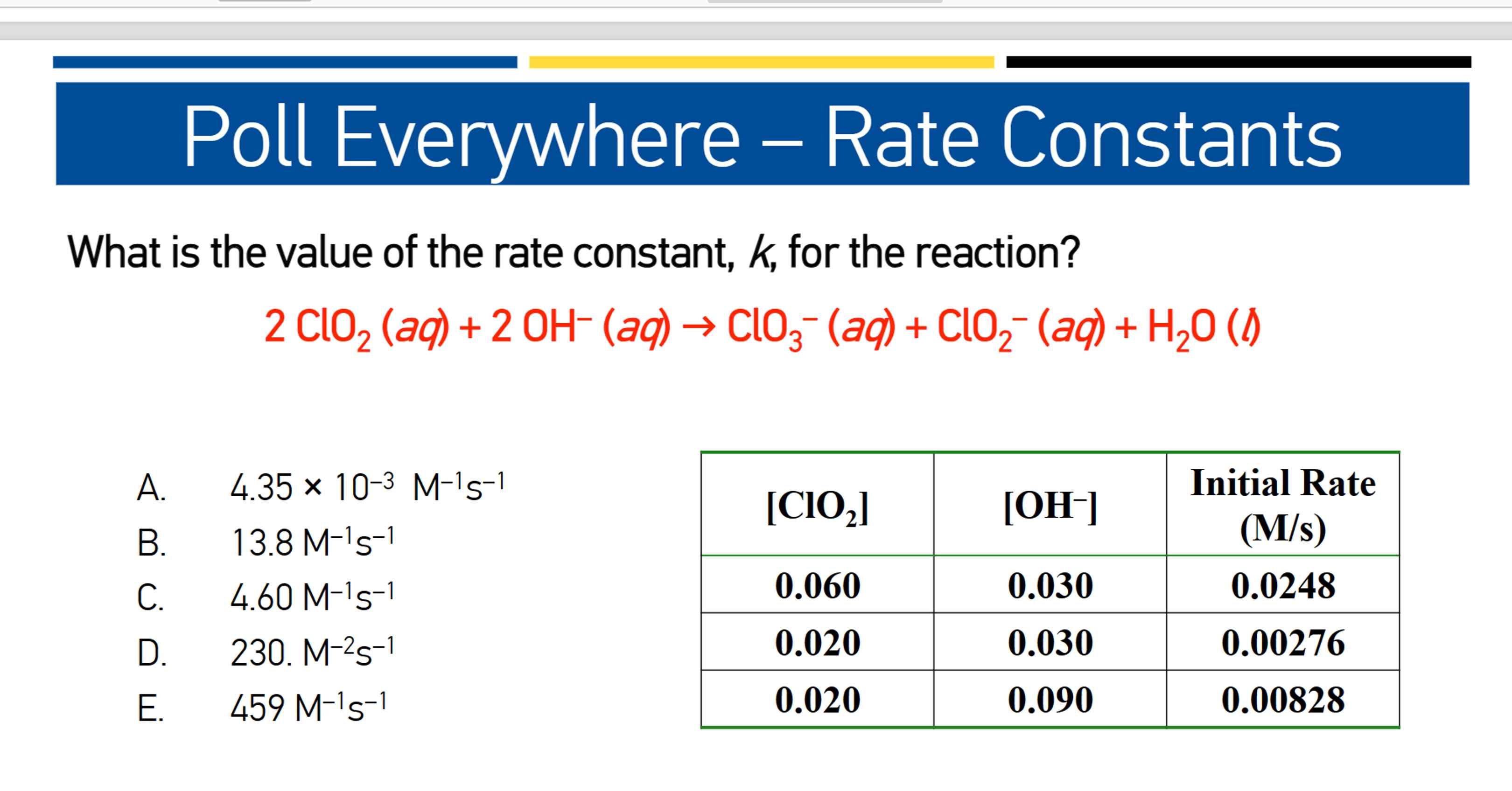
Solved Question Chegg Determine the fourier cosine series of the even triangle function represented by fig. \(\pageindex{1}\). solution. view tutorial on . the triangle function depicted in fig. \(\pageindex{1}\) is an even function of \(x\) with period \(2\pi\) (i.e., \(l =\pi\)). its definition on \(0 < x <\pi\) is given by \[f(x)=1 \frac{2x}{\pi}.\nonumber\]. 0 2 in the fourier series. this allows us to represent functions that are, for example, entirely above the x−axis. with a sufficient number of harmonics included, our ap proximate series can exactly represent a given function f(x) f(x) = a 0 2 a 1 cosx a 2 cos2x a 3 cos3x b 1 sinx b 2 sin2x b 3 sin3x toc jj ii j i back. Series test says that the series converges. to see that the series does not converge absolutely, it suffices to show that the series x∞ n=0 (−1) n √ 1 n2 1 = x∞ n=0 1 √ n2 1 diverges. to see this, do a limit comparison with the divergent series p 1 n: lim n→∞ √ 1 n2 1 1 n = lim n→∞ n √ n2 1 = lim n→∞ 1 √n n n2 1. This section explains three fourier series: sines, cosines, and exponentials eikx. square waves (1 or 0 or −1) are great examples, with delta functions in the derivative. we look at a spike, a step function, and a ramp—and smoother functions too. Determine if the series \( \displaystyle \sum\limits {n = 0}^\infty {{a n}} \) is convergent or divergent. if the series is convergent determine the value of the series. \(\displaystyle {s n} = \frac{{5 8{n^2}}}{{2 7{n^2}}}\) solution. The value of the power series (for our purposes) is that it provides ‘local’ approximations to a function near x 0. for instance, suppose we have a power series for f(x) around zero: f(x) = x1 n=0 a nx n: de ne, for m= 0;1;2; , p m(x) = xm n=0 a nx n 3.
Solve Chegg Series test says that the series converges. to see that the series does not converge absolutely, it suffices to show that the series x∞ n=0 (−1) n √ 1 n2 1 = x∞ n=0 1 √ n2 1 diverges. to see this, do a limit comparison with the divergent series p 1 n: lim n→∞ √ 1 n2 1 1 n = lim n→∞ n √ n2 1 = lim n→∞ 1 √n n n2 1. This section explains three fourier series: sines, cosines, and exponentials eikx. square waves (1 or 0 or −1) are great examples, with delta functions in the derivative. we look at a spike, a step function, and a ramp—and smoother functions too. Determine if the series \( \displaystyle \sum\limits {n = 0}^\infty {{a n}} \) is convergent or divergent. if the series is convergent determine the value of the series. \(\displaystyle {s n} = \frac{{5 8{n^2}}}{{2 7{n^2}}}\) solution. The value of the power series (for our purposes) is that it provides ‘local’ approximations to a function near x 0. for instance, suppose we have a power series for f(x) around zero: f(x) = x1 n=0 a nx n: de ne, for m= 0;1;2; , p m(x) = xm n=0 a nx n 3.

Solve Chegg Determine if the series \( \displaystyle \sum\limits {n = 0}^\infty {{a n}} \) is convergent or divergent. if the series is convergent determine the value of the series. \(\displaystyle {s n} = \frac{{5 8{n^2}}}{{2 7{n^2}}}\) solution. The value of the power series (for our purposes) is that it provides ‘local’ approximations to a function near x 0. for instance, suppose we have a power series for f(x) around zero: f(x) = x1 n=0 a nx n: de ne, for m= 0;1;2; , p m(x) = xm n=0 a nx n 3.
