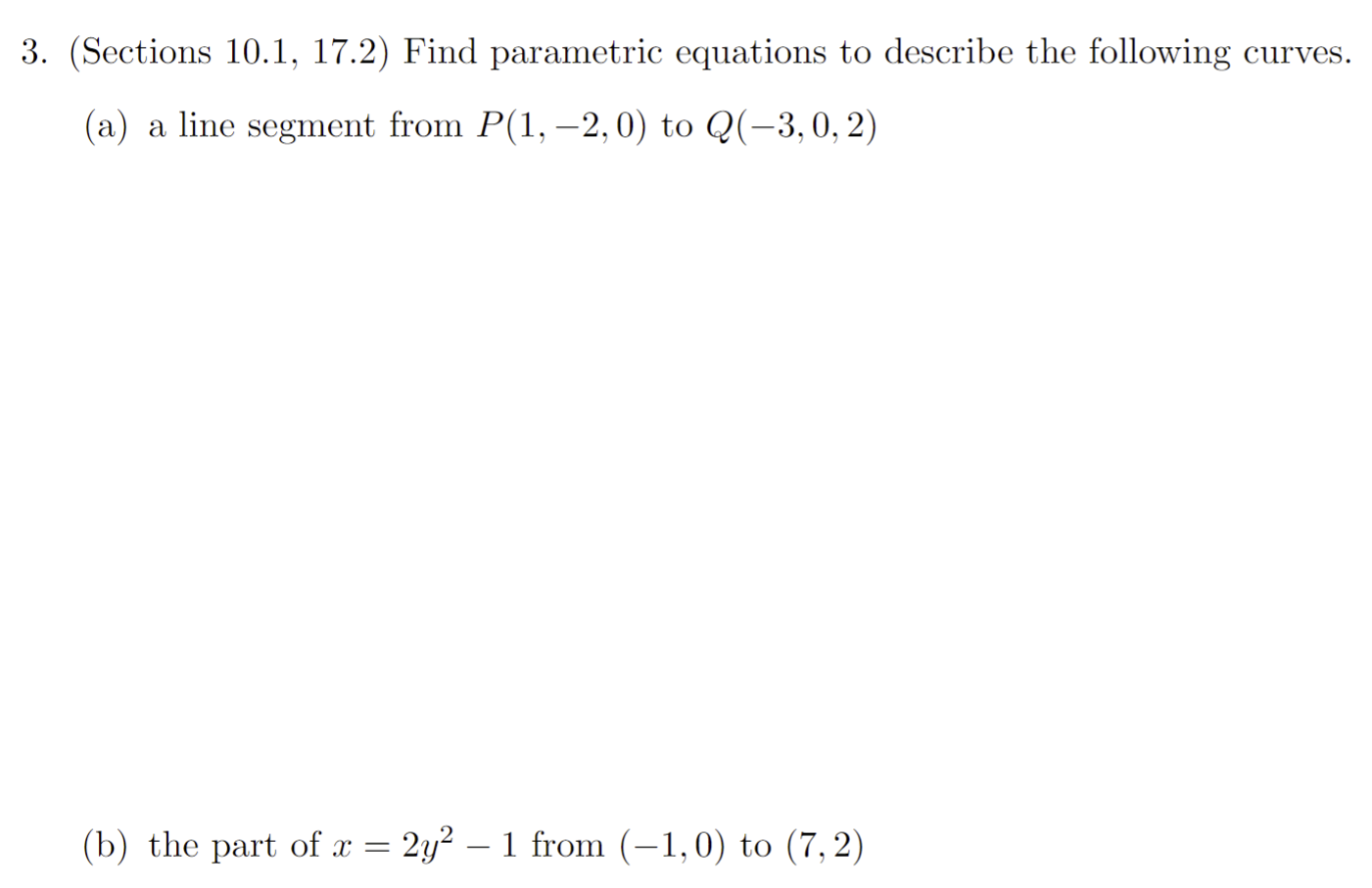
Solved Sections 10 1 17 2 ï Find Parametric Equations To Chegg Question: in this sections, we would like to explore parametric equations with calculus: dy = dy dy dt dx dx 1) we use the formula dt to find the derivative of y with respect to x. however, for the second derivative dy? dt2 dx2 d2x dt2 is not correct!!!!!. The position of a particle is given by the parametric equations x = sin(t) and y = cos(t) where f represents time. we know that the shape of the path of the particle is a circle. a) how long does it take the particle to go once around the circle?.

Solved Homework Parametric Equations 1 Find Parametric Chegg What is the method to find the parametric equations for all types of parabolas (in both directions)? so if i had 2 2 points then: parametrise from a a to b b where a = (3 2√, 9) a = (3 2, 9) and b = (0, 0) b = (0, 0). what would x(t) x (t) and y(t) y (t) be? is there a general way to solve all parabolic parametric equations? if yes, then how?. In this exercise, we explore why parametric equations with two parameters typically graph as surfaces. if x=f(u, v), y=g(u, v) and z=h(u, v), substitute in a constant v = v 1 v=v {1} v = v 1 and let c 1 c 1 c 1 be the curve with parametric equations x = f ( u , v 1 ) x=f\left(u, v {1}\right) x = f ( u , v 1 ) , y = g ( u , v 1 ) y=g\left(u, v. Parametric equations are those that define rectangular equations in the context of a single parameter. use provided examples to evaluate several parametric equations to see how they can be. We would like to understand how a parametric equation represents a curve and distinguish some small differences among similar parametric equations: 1) for the following parametric equations, use a table (shown below) t х у of values of t, x and y to find several points of the curve and then sketch the curve: i) *= t, y=t 6, 4

Solved In Exploration 6 2 2 Working With Parametric Chegg Parametric equations are those that define rectangular equations in the context of a single parameter. use provided examples to evaluate several parametric equations to see how they can be. We would like to understand how a parametric equation represents a curve and distinguish some small differences among similar parametric equations: 1) for the following parametric equations, use a table (shown below) t х у of values of t, x and y to find several points of the curve and then sketch the curve: i) *= t, y=t 6, 4

Solved Using Parametric Equations In Exercises Chegg Parametric equations and curves – in this section we will introduce parametric equations and parametric curves (i.e. graphs of parametric equations). we will graph several sets of parametric equations and discuss how to eliminate the parameter to get an algebraic equation which will often help with the graphing process. Search our library of 100m curated solutions that break down your toughest questions. ask one of our real, verified subject matter experts for extra support on complex concepts. test your knowledge anytime with practice questions. create flashcards from your questions to quiz yourself. Parametric integration can be a tricky topic to grasp, so let's walk through a couple of examples to solidify our understanding. suppose we have a parametrically defined curve with ( x (t) = 2 t ) and ( y (t) = e^t 1 ). our goal is to determine the enclosed area between the x axis, the line ( x = 0 ), and the given curve. Eliminate the parameter θ to obtain a cartesian equation for each of the following parametric expressions. find the x and y intercepts for each pair of parametric equations. x = 4 at 2 , y = a ( 2 t 1 ) , t ∈ . where a is non zero constant. given the curves passes through the point a ( 4,0 ) , find the value of a .

Question Chegg Parametric integration can be a tricky topic to grasp, so let's walk through a couple of examples to solidify our understanding. suppose we have a parametrically defined curve with ( x (t) = 2 t ) and ( y (t) = e^t 1 ). our goal is to determine the enclosed area between the x axis, the line ( x = 0 ), and the given curve. Eliminate the parameter θ to obtain a cartesian equation for each of the following parametric expressions. find the x and y intercepts for each pair of parametric equations. x = 4 at 2 , y = a ( 2 t 1 ) , t ∈ . where a is non zero constant. given the curves passes through the point a ( 4,0 ) , find the value of a .

Solved Consider The Following Parametric Equations Complete Chegg
