Solved Let A 1 3 7 2 0 1 2 7 1 3 2 4 9 5 1 3 6 Chegg

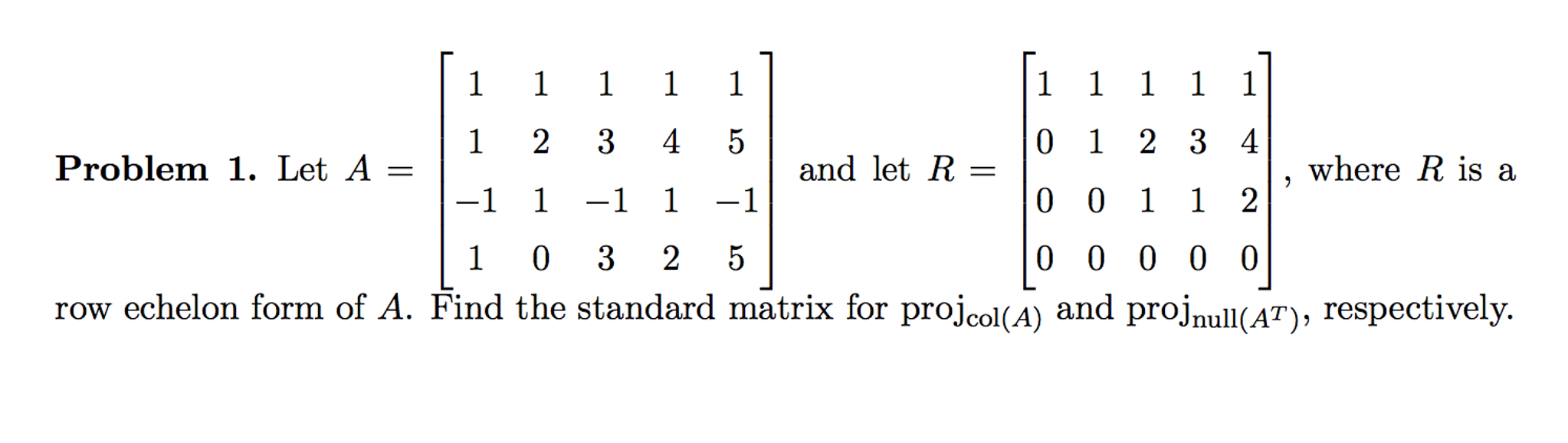
Solved Let A 1 1 1 1 1 1 2 3 4 5 1 1 1 1 1 1 0 3 2 Chegg Let a = [ 1 3 7 2 0 1 2 7 1 3 2 4 9 5 1 3 6 11 9 1] and b = [1 3 7 2 0 0 1 0 1 3 0 0 5 3 5 0 0 0 0 0]. it can be shown that matrix a is row equivalent to matrix b. find a basis for col a. [ 1 1 2 3], [3 2 4 6], [2 1 5 9] [1 0 0 0], [ 3 1 0 0], [ 7 0 5 0] [ 1 1 2 3], [3 2 4 6], [7 7 9 11], [2 1 5 9], [0 3 1 1]. Free equation solver helps you to calculate linear, quadratic and polynomial systems of equations. answers, graphs, roots, alternate forms.

Solved Let A 1 3 7 2 0 1 2 7 1 3 2 4 9 5 1 3 6 Chegg Free math problem solver answers your linear algebra homework questions with step by step explanations. Latest solved 3 x 5 = 25 3 x 2 x 2 55 x 87 = 0 graph of a circle with radius 10 and center at (15, 3) 2, 9, 16, 23, 30 0.15 (y 0.2) = 2 0.5 (1 y) find the midpoint of the points (1,2) and (3, 4) find the domain and range of (7,3), ( 2, 2), (4,1), (4,1), ( 9,0) (0,7) 7, 15, 23, 31, 39, 47, 55, 63 0.003234 to scientific 1, 7, 13, 19, 25, 31, 37, 43 lcm (26,14,91) d i s tan c e (3, 8) (5, 1. Two new drugs are to be tested using a group of 50 laboratory mice, each tagged with a number for identification purposes. drug a is to be given to 17 mice, drug b is to be given to another 17 mice, and the remaining 16 mice are to be used as controls. how many ways can the assignment of treatments to mice be made?. Concept: calculation: given, a = {1, 3, 7, 9, 11} and b = {2, 4, 5, 7, 8, 10, 12}. f : a → b according to the question, f (1) f (3) = 14 possible cases are: (i) 2 12 (ii) 4 10 ∴ onto functions = 2 × (2 × 5 × 4 × 3) = 240 ∴ number of onto functions = 240. the correct answer is option 4. download solution pdf share on whatsapp.

Solved Let A 2 1 1 1 0 1 2 2 2 0 1 1 2 2 1 0 Chegg Two new drugs are to be tested using a group of 50 laboratory mice, each tagged with a number for identification purposes. drug a is to be given to 17 mice, drug b is to be given to another 17 mice, and the remaining 16 mice are to be used as controls. how many ways can the assignment of treatments to mice be made?. Concept: calculation: given, a = {1, 3, 7, 9, 11} and b = {2, 4, 5, 7, 8, 10, 12}. f : a → b according to the question, f (1) f (3) = 14 possible cases are: (i) 2 12 (ii) 4 10 ∴ onto functions = 2 × (2 × 5 × 4 × 3) = 240 ∴ number of onto functions = 240. the correct answer is option 4. download solution pdf share on whatsapp. Your solution’s ready to go! our expert help has broken down your problem into an easy to learn solution you can count on. there are 2 steps to solve this one. not the question you’re looking for? post any question and get expert help quickly. Problem 2.2.1 from smith eggen's a transition to advanced mathematics 7th edition from chapter 2, set theory set operations enjoy, and i am available for tutoring and private classes!. Solutions for over 200 topics within math, chemistry and physics problems across all levels of education, from elementary school to college. see step by step solutions for doing basic multiplication or division problems: see the steps for applying the order of operations for arithmetic:. Define the three layers as domain $x$, codomain $f (x)$, and codomain $f (f (x))$. each one of them is contained in the set $a$. we know that $f (f (x))$ is a constant function, or in other words, can only take on one value. so, we can start off by choosing that value $c$ in $7$ ways.

Solved Let A 1 2 1 1 1 4 0 3 2 3 0 1 2 1 6 3 Chegg Your solution’s ready to go! our expert help has broken down your problem into an easy to learn solution you can count on. there are 2 steps to solve this one. not the question you’re looking for? post any question and get expert help quickly. Problem 2.2.1 from smith eggen's a transition to advanced mathematics 7th edition from chapter 2, set theory set operations enjoy, and i am available for tutoring and private classes!. Solutions for over 200 topics within math, chemistry and physics problems across all levels of education, from elementary school to college. see step by step solutions for doing basic multiplication or division problems: see the steps for applying the order of operations for arithmetic:. Define the three layers as domain $x$, codomain $f (x)$, and codomain $f (f (x))$. each one of them is contained in the set $a$. we know that $f (f (x))$ is a constant function, or in other words, can only take on one value. so, we can start off by choosing that value $c$ in $7$ ways.

Solved Let рќђґ 1 в 3 0 в 4 1 1 рќђµ 0 1 1 0 в 1 1 Chegg Solutions for over 200 topics within math, chemistry and physics problems across all levels of education, from elementary school to college. see step by step solutions for doing basic multiplication or division problems: see the steps for applying the order of operations for arithmetic:. Define the three layers as domain $x$, codomain $f (x)$, and codomain $f (f (x))$. each one of them is contained in the set $a$. we know that $f (f (x))$ is a constant function, or in other words, can only take on one value. so, we can start off by choosing that value $c$ in $7$ ways.