Solved Problem 7 Let F A B And G B C Be Functions Chegg (a) prove that if f and g are injective (i.e. one to one), then so is g f . (b) prove that if g f is injective, then f is injective. your solution’s ready to go! our expert help has broken down your problem into an easy to learn solution you can count on. question: let f : a → b and g : b → c be functions. G : b → c, if f and g are injective, then g f is also injective. we can prove this with a direct proof, by being systematic about using our definitions and standard proof outlines.

Solved 1 Suppose That F A B And G B C Prove The Chegg Suppose that f: a → b f: a → b and g: b → c g: b → c are functions. prove the following: (a) if g ∘ f g ∘ f is injective, then f f is injective. proof. assume that f f is not injective. then there exists a, b ∈ a a, b ∈ a such that f(a) = f(b) f (a) = f (b) and a ≠ b a ≠ b. Exercise 0.3.10: let f : a b and g: b c be functions: prove that if go f is injective, then f is injective. b) prove that if g o f is surjective, then g is surjective. find an explicit example where g o f is bijective, but neither f nor g is bijective. submitted by katie w. oct. 06, 2021 04:06 a.m. Step 1: let's assume that ₁ ₂ g ∘ f (a ₁) = g ∘ f (a ₂) for some elements ₁ ₂ a ₁ and a ₂ in a. step 2: apply the definition of the composition f. Theorem 4.6.9 a function f: a → b f: a → b has an inverse if and only if it is bijective. proof. suppose g g is an inverse for f f (we are proving the implication ⇒ ⇒). since g ∘ f =ia g ∘ f = i a is injective, so is f f (by 4.4.1 (a)). since f ∘ g =ib f ∘ g = i b is surjective, so is f f (by 4.4.1 (b)).

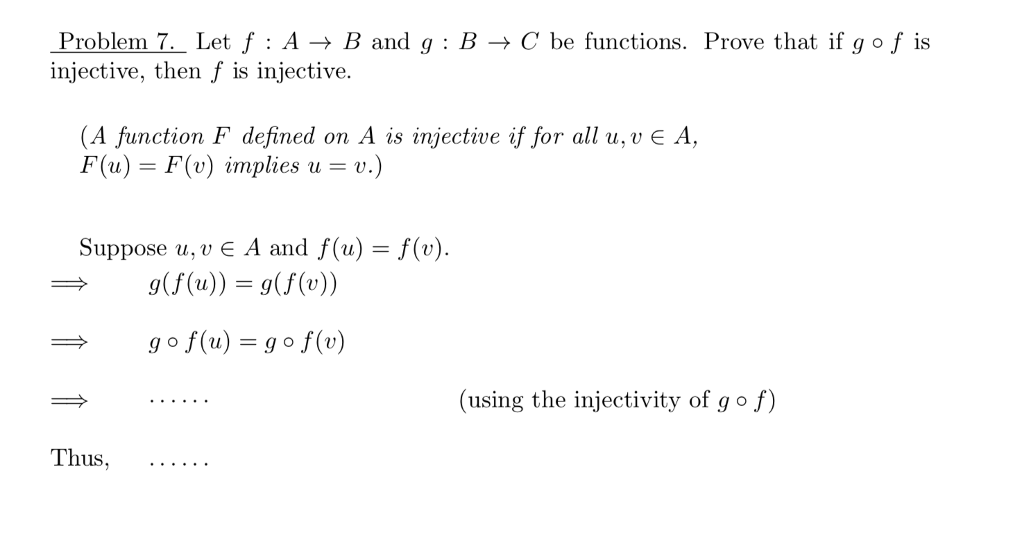
Solved 7 Let F A B And G B C Be Functions Prove That If Chegg Step 1: let's assume that ₁ ₂ g ∘ f (a ₁) = g ∘ f (a ₂) for some elements ₁ ₂ a ₁ and a ₂ in a. step 2: apply the definition of the composition f. Theorem 4.6.9 a function f: a → b f: a → b has an inverse if and only if it is bijective. proof. suppose g g is an inverse for f f (we are proving the implication ⇒ ⇒). since g ∘ f =ia g ∘ f = i a is injective, so is f f (by 4.4.1 (a)). since f ∘ g =ib f ∘ g = i b is surjective, so is f f (by 4.4.1 (b)). Given that f and g are injective functions, meaning that for any two elements in their respective domains, they map to different elements in their respective codomains. Theorem 4.4.1 suppose f: a → b f: a → b and g: b → c g: b → c are functions. a) if g ∘ f g ∘ f is injective then f f is injective. b) if g ∘ f g ∘ f is surjective then g g is surjective. proof. we prove part (a), leaving part (b) as an exercise. suppose a,a′ ∈ a a, a ′ ∈ a and f(a) = f(a′) f (a) = f (a ′). we wish to prove a =a′ a = a ′. we have. Theorem 4.3.5 if f: a → b f: a → b and g: b → c g: b → c are injective functions, then g ∘ f: a → c g ∘ f: a → c is injective also. proof. suppose g(f(a)) = g(f(a′)) g (f (a)) = g (f (a ′)). since g g is injective, f(a) = f(a′) f (a) = f (a ′). since f f is injective, a =a′ a = a ′. Let f : a → b and g : b → c be functions. prove that if g f is injective, then f is.

Solved Problem 4 Prove The Theorem Theorem If F A B Is Chegg Given that f and g are injective functions, meaning that for any two elements in their respective domains, they map to different elements in their respective codomains. Theorem 4.4.1 suppose f: a → b f: a → b and g: b → c g: b → c are functions. a) if g ∘ f g ∘ f is injective then f f is injective. b) if g ∘ f g ∘ f is surjective then g g is surjective. proof. we prove part (a), leaving part (b) as an exercise. suppose a,a′ ∈ a a, a ′ ∈ a and f(a) = f(a′) f (a) = f (a ′). we wish to prove a =a′ a = a ′. we have. Theorem 4.3.5 if f: a → b f: a → b and g: b → c g: b → c are injective functions, then g ∘ f: a → c g ∘ f: a → c is injective also. proof. suppose g(f(a)) = g(f(a′)) g (f (a)) = g (f (a ′)). since g g is injective, f(a) = f(a′) f (a) = f (a ′). since f f is injective, a =a′ a = a ′. Let f : a → b and g : b → c be functions. prove that if g f is injective, then f is.
