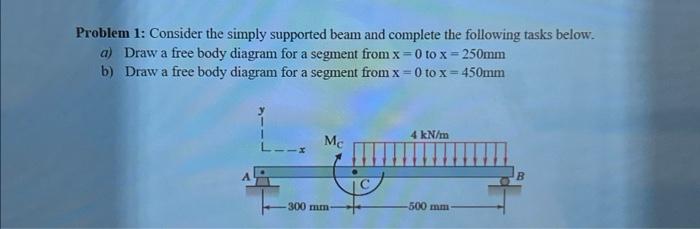
Solved Problem 1 Consider The Simply Supported Beam And Problem 2: consider the simply supported beam below and complete the following tasks a) draw a free body diagram for a segment from a point a to a point between points b and c. To solve these by force method superposition you can treat each of these problems as two simply supported beams that have compatible slope where they are joined together at b b. one beam has flexural rigidity e i e i, and the other has flexural rigidity 2 e i 2e i.

1 For The Simply Supported Beam Solved In Problem 2 Chegg Q 4.3 consider the simply supported t beam shown in the figure below. the live load is assumed to be 600 plf. the followin. Q the rectangular beam, with cross sectional width b, height h, young's modulus erect, has the same flexural rigidity as a. The simply supported beam as shown in figure below carries two concentrated loads. a) derive the expressions for the shear force and the bending moment for each segment of the beam. b) sketch the shear force and bending moment diagrams. There are 3 steps to solve this one. a) here ra is reaction force developed at a and v (x) is shear force acting at a distance x from poin not the question you’re looking for? post any question and get expert help quickly.

Solved Problem 1 Consider The Simply Supported Beam In Chegg The simply supported beam as shown in figure below carries two concentrated loads. a) derive the expressions for the shear force and the bending moment for each segment of the beam. b) sketch the shear force and bending moment diagrams. There are 3 steps to solve this one. a) here ra is reaction force developed at a and v (x) is shear force acting at a distance x from poin not the question you’re looking for? post any question and get expert help quickly. The document provides solutions to problems involving the design of reinforced concrete beams. problem 2.1 calculates the required steel area for a beam with given dimensions and material properties. problem 2.2 calculates required steel areas for beams carrying different factored moments. For a simply supported beam of length l, the bending moment m is described as m = a(x x3 l2), 0 ≤ x < l; where a is a constant. the shear force will be zero at q9. Sample problems include determining stress in a cantilever beam carrying a uniformly distributed load, a simply supported beam with a concentrated load, and minimum beam height needed to limit stress to a specified value. The main types of beams covered are simply supported, cantilever, overhanging, continuous, and propped cantilever beams. it provides examples of calculating the support reactions of simply supported, cantilever, and continuous beams using free body diagrams and the equations of static equilibrium.

Solved Problem 2 Consider The Simply Supported Beam Below Chegg The document provides solutions to problems involving the design of reinforced concrete beams. problem 2.1 calculates the required steel area for a beam with given dimensions and material properties. problem 2.2 calculates required steel areas for beams carrying different factored moments. For a simply supported beam of length l, the bending moment m is described as m = a(x x3 l2), 0 ≤ x < l; where a is a constant. the shear force will be zero at q9. Sample problems include determining stress in a cantilever beam carrying a uniformly distributed load, a simply supported beam with a concentrated load, and minimum beam height needed to limit stress to a specified value. The main types of beams covered are simply supported, cantilever, overhanging, continuous, and propped cantilever beams. it provides examples of calculating the support reactions of simply supported, cantilever, and continuous beams using free body diagrams and the equations of static equilibrium.

Solved Problem 2 Consider The Simply Supported Beam Below Chegg Sample problems include determining stress in a cantilever beam carrying a uniformly distributed load, a simply supported beam with a concentrated load, and minimum beam height needed to limit stress to a specified value. The main types of beams covered are simply supported, cantilever, overhanging, continuous, and propped cantilever beams. it provides examples of calculating the support reactions of simply supported, cantilever, and continuous beams using free body diagrams and the equations of static equilibrium.
