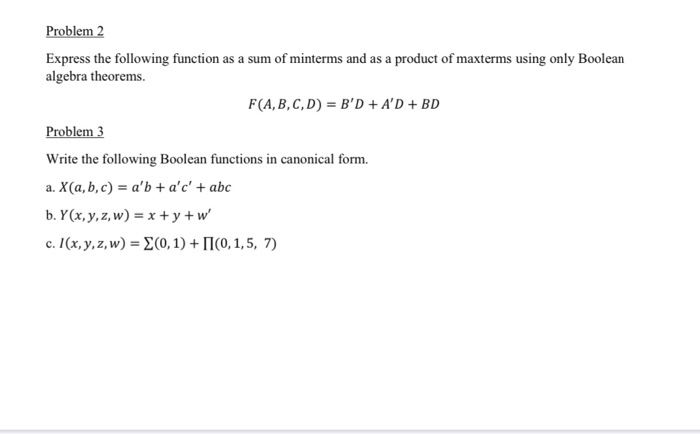
Solved Problem 2 Express The Following Function As A Sum Of Chegg There are 2 steps to solve this one. problem 2 express the following function as a sum of minterms and as a product of maxterms using only boolean algebra theorems. f (a,b,c,d) = b'd a'd bd problem 3 write the following boolean functions in canonical form. a. x (a,b,c) = a'b a'd' abc b. If function f(x, y, z) = ∑ m(2, 3, 4, 5) is implemented using sop form, the resultant boolean function would be .

Solved 4 Express The Following Function As A Chegg Given x(0) = x'(0) = 0, we can solve for a and b. substituting t = 0 into x(t) and x'(t), we find a = 0 and b = 0. therefore, the solution of the initial value problem is: this is a single oscillation with period 2π 6 = π 3. to graph this function, plot y = 13 21 sin(6t) for 0 ≤ t ≤ 2π. Free math problem solver answers your algebra homework questions with step by step explanations. Solution: the function is defined in terms of its minterms. to find a pos expression we should start with the definition in terms of maxterms, which isf =Πm(1,3,9,11,13). thus, f = m1 ·m3 · m9 · m11 ·m13 =(x1 x2 x3 x4)(x1 x2 x3 x4)(x1 x2 x3 x4)(x1 x2 x3 x4)(x1 x2 x3 x4) we can rewrite the product of the first two maxterms as. B) directly write the product of maxterms in ∏ format from the sum of minterms in the ∑ format obtained in previous part. here’s the best way to solve it. solution.
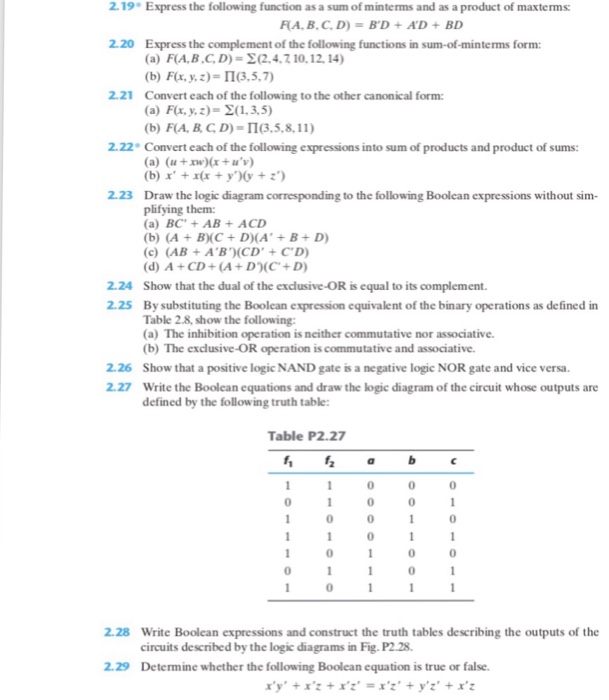
Solved 2 19 Express The Following Function As A Sum Of Chegg Solution: the function is defined in terms of its minterms. to find a pos expression we should start with the definition in terms of maxterms, which isf =Πm(1,3,9,11,13). thus, f = m1 ·m3 · m9 · m11 ·m13 =(x1 x2 x3 x4)(x1 x2 x3 x4)(x1 x2 x3 x4)(x1 x2 x3 x4)(x1 x2 x3 x4) we can rewrite the product of the first two maxterms as. B) directly write the product of maxterms in ∏ format from the sum of minterms in the ∑ format obtained in previous part. here’s the best way to solve it. solution. The function f (a, b, c, d) = b ′ d a ′ d b d can be expressed as a sum of minterms: f (a, b, c, d) = m 0 m 6 m 8 m 13 and as a product of maxterms. maxterms would include combinations yielding a result of zero based on our evaluations. In this problem we guide you through a simple self contained proof that f is log concave. recall that f is log concave if and only if f′′(x)f(x) ≤ f′(x)2 for all x. verify that f′′(x)f(x) f′(x)2 for x 0. that leaves us the hard part, which is ≤ to show the inequality for x < ≥. 0. verify that for any t and x we have t2 2 ≥ −x2 2 xt. Obtain the truth table of the function. draw the logic diagram using the original boolean expression. simplify the function to a minimim number of literals using boolean algebra. the inhibition operation is neither commutative nor associative. the exclusive or operation is commutative and associative. Everywhere. a calculus theorem tells us that if two functions are each differentiable at a point, then their sum is differentiable at that point and the sum of the derivatives is the derivative of the sum. therefore f g must be differentiable everywhere and satisfy (f g)0 = f0 g0. from the fact that f and g belong to w we also know that.

Solved Express The Following Function As A Sum Of Minterms Chegg The function f (a, b, c, d) = b ′ d a ′ d b d can be expressed as a sum of minterms: f (a, b, c, d) = m 0 m 6 m 8 m 13 and as a product of maxterms. maxterms would include combinations yielding a result of zero based on our evaluations. In this problem we guide you through a simple self contained proof that f is log concave. recall that f is log concave if and only if f′′(x)f(x) ≤ f′(x)2 for all x. verify that f′′(x)f(x) f′(x)2 for x 0. that leaves us the hard part, which is ≤ to show the inequality for x < ≥. 0. verify that for any t and x we have t2 2 ≥ −x2 2 xt. Obtain the truth table of the function. draw the logic diagram using the original boolean expression. simplify the function to a minimim number of literals using boolean algebra. the inhibition operation is neither commutative nor associative. the exclusive or operation is commutative and associative. Everywhere. a calculus theorem tells us that if two functions are each differentiable at a point, then their sum is differentiable at that point and the sum of the derivatives is the derivative of the sum. therefore f g must be differentiable everywhere and satisfy (f g)0 = f0 g0. from the fact that f and g belong to w we also know that.

Solved Problem 11 Evaluate The Following Sum Chegg Obtain the truth table of the function. draw the logic diagram using the original boolean expression. simplify the function to a minimim number of literals using boolean algebra. the inhibition operation is neither commutative nor associative. the exclusive or operation is commutative and associative. Everywhere. a calculus theorem tells us that if two functions are each differentiable at a point, then their sum is differentiable at that point and the sum of the derivatives is the derivative of the sum. therefore f g must be differentiable everywhere and satisfy (f g)0 = f0 g0. from the fact that f and g belong to w we also know that.

Solved Hello Please Help Me Solve This Problem Using The Chegg
