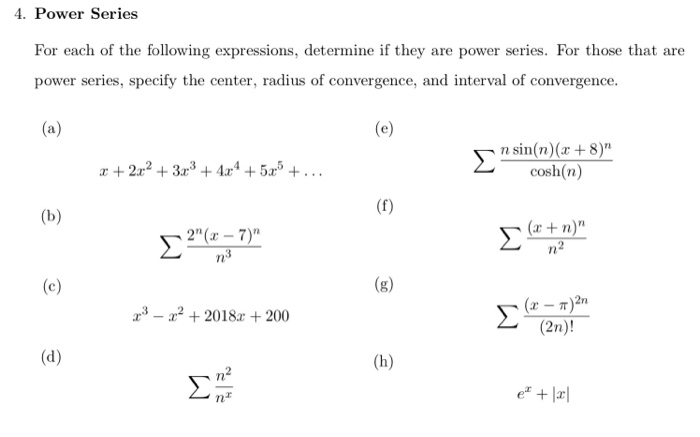
Solved 4 Power Series For Each Of The Following Chegg Our expert help has broken down your problem into an easy to learn solution you can count on. please help on part a, b, and c! there are 3 steps to solve this one. Power series for each of the following expressions, determine if they are power series. for those that are power series, specify the center, radius of convergence, and interval of convergence n sin(n)(x 8)" cosh(n) 2a2 3a3 4r4 5a5 2(7)" 1l r3 a2 2018 200 (2n)!.

Solved Problem 4 For The Following Power Series Determine Chegg For each of the following power series determine the interval and radius of convergence. here is a set of practice problems to accompany the power series section of the series & sequences chapter of the notes for paul dawkins calculus ii course at lamar university. Free online power series calculator find convergence interval of power series step by step. Problem 4 determine the sum of the following power series (you are allowed to use the taylor series for ex and cos(x) we saw in lecture. you don't need to use taylor's inequality to show the convergence). (a) ∑n=0∞(2n!)(−1)n32n(x−4)2n. (b) ∑n=2∞(n−1)!x2n. The value of the power series (for our purposes) is that it provides ‘local’ approximations to a function near x 0. for instance, suppose we have a power series for f(x) around zero: f(x) = x1 n=0 a nx n: de ne, for m= 0;1;2; , p m(x) = xm n=0 a nx n 3.

Solved Problem 3 ï For The Following Power Series Explain Chegg Problem 4 determine the sum of the following power series (you are allowed to use the taylor series for ex and cos(x) we saw in lecture. you don't need to use taylor's inequality to show the convergence). (a) ∑n=0∞(2n!)(−1)n32n(x−4)2n. (b) ∑n=2∞(n−1)!x2n. The value of the power series (for our purposes) is that it provides ‘local’ approximations to a function near x 0. for instance, suppose we have a power series for f(x) around zero: f(x) = x1 n=0 a nx n: de ne, for m= 0;1;2; , p m(x) = xm n=0 a nx n 3. Enhanced with ai, our expert help has broken down your problem into an easy to learn solution you can count on. for each of the following power series determine the interval and radius convergence. here’s the best way to solve it. #### problem 1: the given power series is: $$ \sum\ {n=0}^ {\infty} \frac {6^ {1 n}} { ( 2)^ {3 2n}} (x 4)^n $$. This example demonstrated how we can solve a simple differential equation by first guessing that the solution was in the form of a power series. we would like to explore the use of power series for more general higher order equations. Example 4 determine the radius of convergence and interval of convergence for the following power series. \[\sum\limits {n = 1}^\infty {\frac{{{{\left( {x 6} \right)}^n}}}{{{n^n}}}} \] \[\sum\limits {n = 1}^\infty {\frac{{{{\left( {x 6} \right)}^n}}}{{{n^n}}}} \]. Use a known series to find a power series in x that has the given function as its sum: x sin(x3) ∞ u2n 1 recall the maclaurin series for sin u = (−1)n (2n 1)! ∞ (x3)2n 1 ∞ (x)6n 3 therefore, sin(x3) = (−1)n = (2n 1)! (−1)n . (2n 1)! ∞ (x)6n 4 hence x sin(x3) = (−1)n . (2n 1)! 3.
