
Solved Real Analysis Power Series Prove The Following Chegg By translating, scaling, and reflecting about 0 if necessary, without loss of generality it suffices to prove the following: if on converges then the power series " converges uniformly on the interval (0,1). moreover, since " converges at x = 1, it suffices to show uniform convergence on (0,1). Real analysis: for this particular problem, find the set of all x such that the following power series converge. *please broadly prove and state the definitions. your solution’s ready to go! our expert help has broken down your problem into an easy to learn solution you can count on.

Solved Real Analysis Power Series Prove The Following Chegg I was trying to come up with my own very simple proof of the cauchy criterion for series $\left(\text{i.e., let}\, \{a {n}\}\, \text{be a sequence in}\, \mathbb{r}. \text{the series}\,\sum {n=0}^{\infty}a {n}\, \text{is summable iff}\, \forall \epsilon > 0, \,\exists n \in \mathbb{n}\, \text{such that }\, \forall n > n,\, m \in \mathbb{n. Prove or disprove the following statements. (a) if fn → f uniformly on r, then fn(xn) → f(0) as n → ∞. (b) if fn → f pointwise on r, then fn(xn) → f(0) as n → ∞. solution. • (a) this statement is true. to prove it, we first observe that f is con tinuous since the uniform limit of continuous functions is continuous. • let ϵ. Simplify your answer and compare with the previous example. can you use your results to figure out what function this power series represents? find, with proof, a power series centered at c = 0 for the function f(x) = 1 (1 x) 2, for 1 < x < 1. find a simple expression for n x n and n 2 x n, where |x| < 1. how about n 3 x n. Answer to real analysis(power series): prove the following.

Solved Real Analysis Power Series Prove The Following Chegg Simplify your answer and compare with the previous example. can you use your results to figure out what function this power series represents? find, with proof, a power series centered at c = 0 for the function f(x) = 1 (1 x) 2, for 1 < x < 1. find a simple expression for n x n and n 2 x n, where |x| < 1. how about n 3 x n. Answer to real analysis(power series): prove the following. The value of the power series (for our purposes) is that it provides ‘local’ approximations to a function near x 0. for instance, suppose we have a power series for f(x) around zero: f(x) = x1 n=0 a nx n: de ne, for m= 0;1;2; , p m(x) = xm n=0 a nx n 3. Note. we will prove our theorems for real (a n) but almost all apply equally to real and complex sequences. 13.2 examples (i) a n = 1 : p xn: geometric series : convergent if |x| < 1 divergent if |x| > 1 (ii) a n = 1 n! : p xn n! : exponential series : convergent for all x (iii) a n = 1 n : p ∞ n 1 x n n : logarithmic series : convergent if. In 8.6 we obtained power series representations for certain special functions. in 8.7 we look at the more general problem, namely, which functions have power series representations, and how can we find them? in general: f (n)(a) = 2 · 3 · 4 · · · ncn = n!cn. solving for cn we obtain. cn = n! cn = . n! (x) = (x − a)n n! 1! 2! 3!. Real analysis: for this particular problem, find the set of all x such that the following power series converge. *please broadly prove and state the definitions.

Solved 4 Find A Power Series Representation For The Chegg The value of the power series (for our purposes) is that it provides ‘local’ approximations to a function near x 0. for instance, suppose we have a power series for f(x) around zero: f(x) = x1 n=0 a nx n: de ne, for m= 0;1;2; , p m(x) = xm n=0 a nx n 3. Note. we will prove our theorems for real (a n) but almost all apply equally to real and complex sequences. 13.2 examples (i) a n = 1 : p xn: geometric series : convergent if |x| < 1 divergent if |x| > 1 (ii) a n = 1 n! : p xn n! : exponential series : convergent for all x (iii) a n = 1 n : p ∞ n 1 x n n : logarithmic series : convergent if. In 8.6 we obtained power series representations for certain special functions. in 8.7 we look at the more general problem, namely, which functions have power series representations, and how can we find them? in general: f (n)(a) = 2 · 3 · 4 · · · ncn = n!cn. solving for cn we obtain. cn = n! cn = . n! (x) = (x − a)n n! 1! 2! 3!. Real analysis: for this particular problem, find the set of all x such that the following power series converge. *please broadly prove and state the definitions.
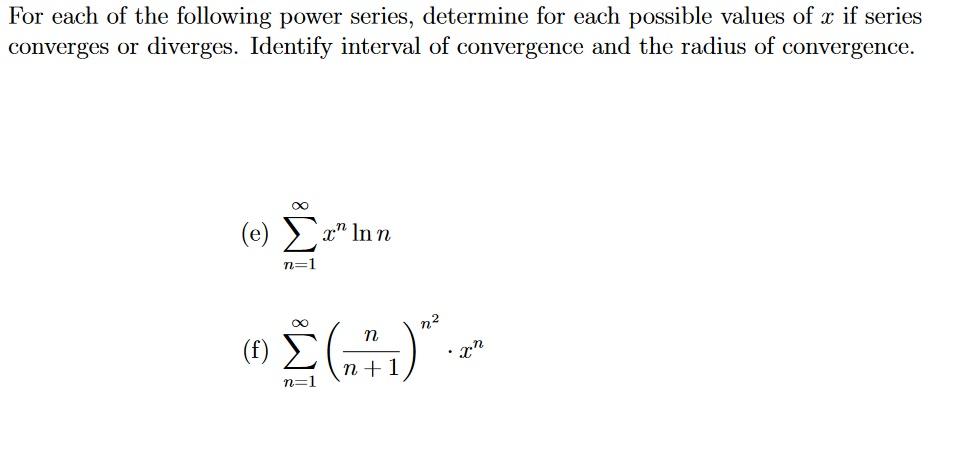
Solved For Each Of The Following Power Series Determine For Chegg In 8.6 we obtained power series representations for certain special functions. in 8.7 we look at the more general problem, namely, which functions have power series representations, and how can we find them? in general: f (n)(a) = 2 · 3 · 4 · · · ncn = n!cn. solving for cn we obtain. cn = n! cn = . n! (x) = (x − a)n n! 1! 2! 3!. Real analysis: for this particular problem, find the set of all x such that the following power series converge. *please broadly prove and state the definitions.
