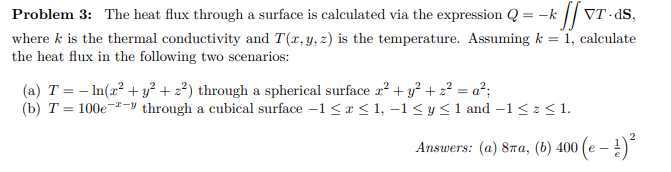
Solved Surface Integrals Please Work Out Problem 3 Part A Chegg Problem 3: the heat flux through a surface is calculated via the expression q=−k∬ ∇t ⋅ds, where k is the thermal conductivity and t (x,y,z) is the temperature. (1) find curl f. (3 points) (2) find ss, curl f. ds. explain your reasoning. (hint: there is no need to evaluate challenging integrals.) (9 points) b) let s be the surface consisting of the cylinder x² y² = 4, the plane x z=6, and the xy plane as shown.

Solved 3 Solve The Following Surface Integrals A Let Chegg Calculate the surface integrals r s r f (x, y, z)ds using the surface given by z = g (x, y): f (x, y, z) = x 2 y 2 z 2 , where g (x, y) = x 2 and x 2 y 2 <= 1. your solution’s ready to go! enhanced with ai, our expert help has broken down your problem into an easy to learn solution you can count on. Here is a set of practice problems to accompany the surface integrals section of the surface integrals chapter of the notes for paul dawkins calculus iii course at lamar university. We now show how to calculate the flux integral, beginning with two surfaces where n and ds are easy to calculate — the cylinder and the sphere. example 1. find the flux of f = z i x j y k outward through the portion of the cylinder x2 y2 = a2 in the first octant and below the plane z = h. solution. the piece of cylinder is pictured. In this section we introduce the idea of a surface integral. with surface integrals we will be integrating over the surface of a solid. in other words, the variables will always be on the surface of the solid and will never come from inside the solid itself.

Solved I Need Help With This Question It Is From Calculus Chegg We now show how to calculate the flux integral, beginning with two surfaces where n and ds are easy to calculate — the cylinder and the sphere. example 1. find the flux of f = z i x j y k outward through the portion of the cylinder x2 y2 = a2 in the first octant and below the plane z = h. solution. the piece of cylinder is pictured. In this section we introduce the idea of a surface integral. with surface integrals we will be integrating over the surface of a solid. in other words, the variables will always be on the surface of the solid and will never come from inside the solid itself. Here are a set of practice problems for the surface integrals chapter of the calculus iii notes. if you’d like a pdf document containing the solutions the download tab above contains links to pdf’s containing the solutions for the full book, chapter and section. 17.6, part 3 surface integrals of scalar functions:evaluate ∬s3ds, where s is the surface with parametric equations x=uv,y=u v, and z=u v,for u2 v2≤1. your solution’s ready to go! our expert help has broken down your problem into an easy to learn solution you can count on. Line and surface integrals: solutions example 5.1 find the work done by the force f(x, y) = x2i − xyj in moving a particle along the curve which runs from (1, 0) to (0, 1) along the unit circle and then from (0, 1) to (0, 0) along the y axis (see figure 5.1). A surface integral is similar to a line integral, except the integration is done over a surface rather than a path. in this sense, surface integrals exp.

Solved 3 Thinking About Surface Integrals Answer The Chegg Here are a set of practice problems for the surface integrals chapter of the calculus iii notes. if you’d like a pdf document containing the solutions the download tab above contains links to pdf’s containing the solutions for the full book, chapter and section. 17.6, part 3 surface integrals of scalar functions:evaluate ∬s3ds, where s is the surface with parametric equations x=uv,y=u v, and z=u v,for u2 v2≤1. your solution’s ready to go! our expert help has broken down your problem into an easy to learn solution you can count on. Line and surface integrals: solutions example 5.1 find the work done by the force f(x, y) = x2i − xyj in moving a particle along the curve which runs from (1, 0) to (0, 1) along the unit circle and then from (0, 1) to (0, 0) along the y axis (see figure 5.1). A surface integral is similar to a line integral, except the integration is done over a surface rather than a path. in this sense, surface integrals exp.
