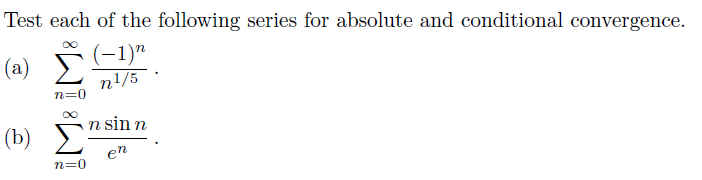
Solved Test Each Of The Following Series For Absolute And Chegg Test each of the following series for absolute convergence, conditional convergence, ordivergence: (a) ∑n=1∞ ( 1)nnn2 5n 1 (b) ∑n=1∞ ( 1)n (n 5)n3 7n 9 (c) ∑n=1∞ (n 12 n2)an where an= ( 1)f (n) and f (n)= [n2]; that is, f (n) is the integerpart of n2. (d) ∑n=1∞ ( 1)nnn 1. your solution’s ready to go!. Test the following series for absolute and conditional convergence: ∑k=3∞ (−1)k log k k log(log k). (1) (1) ∑ k = 3 ∞ (− 1) k log k k log (log k). what test do i have to use to test for convergence of this series? use of the double log always confuses me. i have two more series:.

Solved Series Absolute Test The Following For Convergence Chegg Determine if the series converges absolutely, converges conditionally, or diverges. find the exact value for the sum of the convergent series. $$1 \frac{1}{5} \frac{1}{5^2} \frac{1}{5^3} \frac{1}{5^4} \frac{1}{5^5} \frac{1}{5^6} \frac{1}{5^7} \frac{1}{5^8} $$ i have no clue where to start. Problem 1: compute the absolute error and relative error in approximations of p by p∗. (use calculator!) a) p = π, p∗ =22 7; b) p = π, p∗ =3.1416. solution: for this exercise, you can use either calculator or matlab. a) absolute error: |p−p∗| = |π −22 7| =0.0012645. relative error: |p−p∗| |p| = |π−22 7| π =4.0250×10−4. For each of the following series determine if they are absolutely convergent, conditionally convergent or divergent. here is a set of practice problems to accompany the absolute convergence section of the series & sequences chapter of the notes for paul dawkins calculus ii course at lamar university. Test each of the following series for absolute convergence, conditional convergence, or divergence. show your work and justify your steps by referring to a relevant theorem or result. (a) Σ. your solution’s ready to go! our expert help has broken down your problem into an easy to learn solution you can count on.

Solved 4 10 Points Use Absolute Series Test To Determine Chegg For each of the following series determine if they are absolutely convergent, conditionally convergent or divergent. here is a set of practice problems to accompany the absolute convergence section of the series & sequences chapter of the notes for paul dawkins calculus ii course at lamar university. Test each of the following series for absolute convergence, conditional convergence, or divergence. show your work and justify your steps by referring to a relevant theorem or result. (a) Σ. your solution’s ready to go! our expert help has broken down your problem into an easy to learn solution you can count on. Each of the following statements is an attempt to show that a given series is convergent or divergent using the comparison test (not the limit comparison test.) for each statement, enter c (for "correct") if the argument is valid, or enter i (for "incorrect") if any part of the argument is flawed. Determine whether each series converges absolutely, converges conditionally, or diverges. solution: a {n} = \frac{\ln (n)}{n^{3}}\geq 0 \(\lim {n\rightarrow \infty }= \lim {n\rightarrow \infty }\frac{1}{3n^{3}} = 0\) now, \(\frac{d}{dx}\frac{\ln (x)}{x} = \frac{1 3\lg(x) }{x^{4}} < 0\) therefore, by the alternating series test, the series. Solutions to assignment 6, math 220 1 for each of the following series, indicate whether they converge or diverge and give reasons for your answers. a: p1 k=1 1. 2 . b: p1 k=1k. −k. c: p1 k=2 1 log. d: p1 k=1sin(ˇ=k) e: p1 k=3 1. 2−3 2. f: p1 k=1. k! solution a: converge by comparison to p1 k=1 1. 2. 1–10. choosing convergence tests identify a convergence test for each of the following series. if necessary, explain how to simplify or rewrite the series before applying the convergence test. you do not need to carry out the convergence test.

Solved 1 Test The Following Series For Absolute Or Chegg Each of the following statements is an attempt to show that a given series is convergent or divergent using the comparison test (not the limit comparison test.) for each statement, enter c (for "correct") if the argument is valid, or enter i (for "incorrect") if any part of the argument is flawed. Determine whether each series converges absolutely, converges conditionally, or diverges. solution: a {n} = \frac{\ln (n)}{n^{3}}\geq 0 \(\lim {n\rightarrow \infty }= \lim {n\rightarrow \infty }\frac{1}{3n^{3}} = 0\) now, \(\frac{d}{dx}\frac{\ln (x)}{x} = \frac{1 3\lg(x) }{x^{4}} < 0\) therefore, by the alternating series test, the series. Solutions to assignment 6, math 220 1 for each of the following series, indicate whether they converge or diverge and give reasons for your answers. a: p1 k=1 1. 2 . b: p1 k=1k. −k. c: p1 k=2 1 log. d: p1 k=1sin(ˇ=k) e: p1 k=3 1. 2−3 2. f: p1 k=1. k! solution a: converge by comparison to p1 k=1 1. 2. 1–10. choosing convergence tests identify a convergence test for each of the following series. if necessary, explain how to simplify or rewrite the series before applying the convergence test. you do not need to carry out the convergence test.
