
Solved Two Lines That Do Not Intersect Are Either Or Are Chegg Two lines that do not intersect are either or are coplanar, and are not coplanar. two lines in the same plane are either or in one point. if two lines intersect at a 90 degree angle, then the two lines are two planes that do not intersect are erample 1: identify relationships in space. think of each segment in the picture as part of a line. Skew lines are a pair of lines that are non intersecting, non parallel, and non coplanar. this implies that skew lines can never intersect and are not parallel to each other. given two lines l1:⎣⎡xyz⎦⎤=⎣⎡135⎦⎤ t⎣⎡71−3⎦⎤ and l2:⎣⎡xyz⎦⎤=⎣⎡467⎦⎤ t⎣⎡−102⎦⎤ (a) prove that l1 and l2 are skew lines.
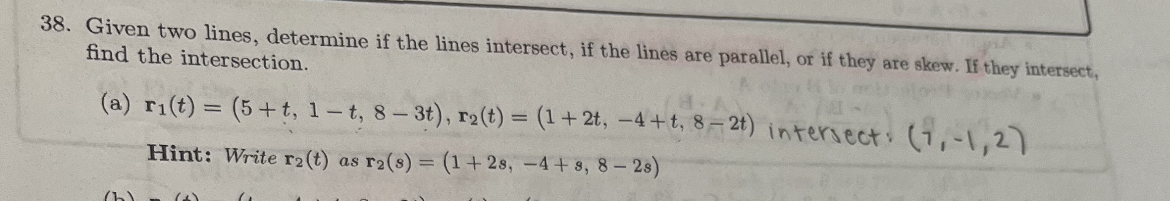
Solved Given Two Lines Determine If The Lines Intersect If Chegg Here’s the best way to solve it. parallel or inconsistent …. Lines are either parallel or intersecting. is only true in the second dimension, in the third dimension, it's planes (entire second dimensions) that intersect and parallel. lines in the third dimension can be "neither", or not intersect or parallel. as @the count mentioned, these lines are called skew. In this tutorial, i will show you how to prove in an exam that two lines are skewed. ⏰timecodes⏰ 00:32 what are skew lines? 00:41 a 3d #geogebra visual representation of skew lines 01:11. In two dimensions, lines are either parallel or they intersect at a single point. if they are parallel, then either they have no points in common, or they share every point in common. in three dimensions, there is a further possibility: a pair of lines might not be parallel and have no points of intersection.

Solved Determine If The Two Lines Intersect And If So Find Chegg In this tutorial, i will show you how to prove in an exam that two lines are skewed. ⏰timecodes⏰ 00:32 what are skew lines? 00:41 a 3d #geogebra visual representation of skew lines 01:11. In two dimensions, lines are either parallel or they intersect at a single point. if they are parallel, then either they have no points in common, or they share every point in common. in three dimensions, there is a further possibility: a pair of lines might not be parallel and have no points of intersection. Study with quizlet and memorize flashcards containing terms like two lines that do not intersect are either or lines, two lines are if they do not intersect and are coplanar, two lines are if they do not intersect and are not coplanar and more. Say you are given two lines: l 1 and l 2 with equations and you are asked to deduce whether or not they intersect. for example: l 1: r 1 = (a 1 ,b 1 ,c 1 ) s(d 1 ,e 1 ,f 1 ). If two lines do not intersect it is possible for them to be non parallel in three dimensional space. in that event the lines are skew. if you are in an elevator, the wall behind you meets the wall to your left in a line segment. similarly, the wall door in front of you meet in a line segment. Depending on the value of the determinant, the two lines either intersect or do not intersect: \(d \neq 0\) if the determinant is non zero, the lines intersect at one point. \(d = 0\) if the determinant is zero, the lines do not intersect. they may be parallel and distinct or coincident.
