
Equations Special Cases One Solution No Solution Infinite Solutions Solve equations that have one solution, no solution, or an infinite number of solutions. solve application problems by using an equation in one variable. when you follow the steps to solve an equation, you try to isolate the variable. you have a solution when you get the equation x = x = some value. This video covers a couple of examples on how to solve linear equations, specifically dealing with special cases. no solution or infinitely many solutions. more.
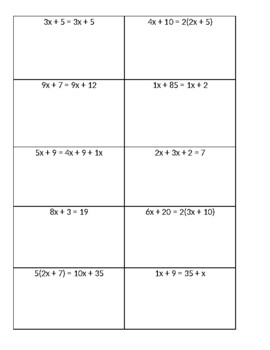
Equations Special Cases One Solution No Solution Infinite Solutions Infinite number of solutions 2) 4) 6) ©o b2s0r1r3j 9khu8tkai qsmoefltdwqazroep 3lclfcb.g 6 4aqlslp jrhi8g8h7thsk mr0eksheernv0endi.l 0 gmcaodke2 uwpiltfhj gigndf0iwnaivtiec yacl7grejber3az n1j.b. This algebra video explains the different between one solution and infinitely many solutions for a linear equation in one variable. special cases of equation. In this section, we will determine the systems that have no solution, and solve the systems that have infinitely many solutions. solve the following system of equations: x y = 7 x y = 9. solution. let us use the gauss jordan method to solve this system. the augmented matrix is. [1 1 | 7 1 1 | 9] [x y = 7 x y = 9]. One, none, or infinite many solutions date period solve each equation. 7(2 5v) = 3v 14 36 7 p = 7(p 5) 5( 2 8m) = 10 5m.

Solving Linear Equations Special Solutions No Solution Infinitely In this section, we will determine the systems that have no solution, and solve the systems that have infinitely many solutions. solve the following system of equations: x y = 7 x y = 9. solution. let us use the gauss jordan method to solve this system. the augmented matrix is. [1 1 | 7 1 1 | 9] [x y = 7 x y = 9]. One, none, or infinite many solutions date period solve each equation. 7(2 5v) = 3v 14 36 7 p = 7(p 5) 5( 2 8m) = 10 5m. In this lesson, you will learn about two different types of equations: equations with infinite solutions and equations with no solution. this video illustrates the lesson material below. watching the video is optional. there are three types of answers you can get when solving for a variable: x = a: where a represents all real numbers. There are three cases that can come up as we are solving linear equations. we have already seen one, where an equation has one solution. sometimes we come across equations that don’t have any solutions, and even some that have an infinite number of solutions. the case where an equation has no solution is illustrated in the next examples. Solving system of equations special cases a set of linear equations with the same two variables is called a system of linear equations. the system of linear equations can have the following solutions. (i) unique solutions (ii) no solution (iii) infinitely many solution. · solve equations that have one solution, no solution, or an infinite number of solutions. · solve application problems by using an equation in one variable. when you follow the steps to solve an equation, you try to isolate the variable. you have a solution when you get the equation x = some value.
