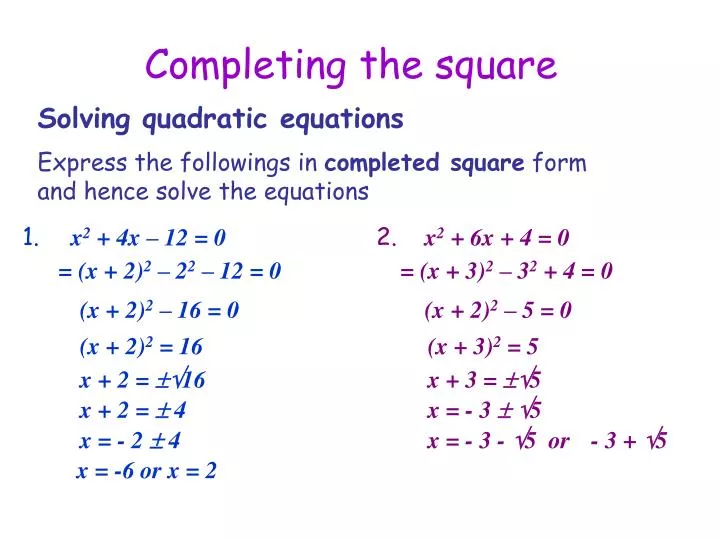
Solving Quadratic Equations By Completing The Square Ppt Tessshebaylo Solving general quadratic equations by completing the square. we can complete the square to solve a quadratic equation (find where it is equal to zero). but a general quadratic equation may have a coefficient of a in front of x 2: ax 2 bx c = 0. to deal with that we divide the whole equation by "a" first, then carry on: x 2 (b a)x c a. Learn how to solve the quadratic equations by the method of completing the square with formula, steps, examples, and diagram.
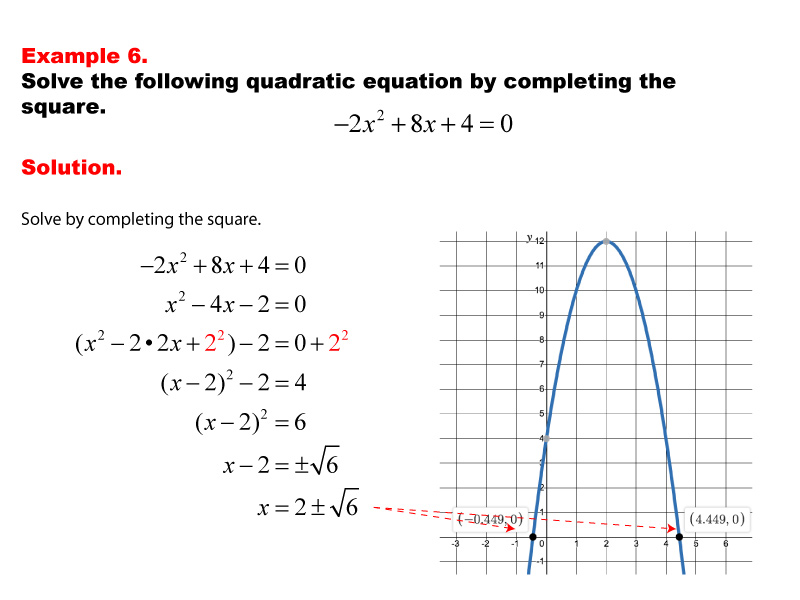
Student Tutorial Solving Quadratic Equations In Standard Form By Example 2: solve the quadratic equation below using the method of completing the square. solution: subtract [latex]2[ latex] from both sides of the quadratic equation to eliminate the constant on the left side. One option is to change a quadratic equation into a perfect square trinomial by using a procedure called completing the square. the following table shows examples of perfect square trinomials in different forms. Let’s practice the questions on completing the square method of solving quadratic equations. find the roots of 4x 2 3x 5 = 0 by the method of completing the square. find the roots of the equation 5x 2 – 6x – 2 = 0 by the method of completing the square. find two consecutive odd positive integers, the sum of whose squares is 290. For quadratic equations that cannot be solved by factorising, we use a method which can solve all quadratic equations called completing the square. we use this later when studying circles in plane analytic geometry. completing the square comes from considering the special formulas that we met in square of a sum and square of a difference earlier:.

Solving Quadratic Equations By Completing The Square A Plus Topper Let’s practice the questions on completing the square method of solving quadratic equations. find the roots of 4x 2 3x 5 = 0 by the method of completing the square. find the roots of the equation 5x 2 – 6x – 2 = 0 by the method of completing the square. find two consecutive odd positive integers, the sum of whose squares is 290. For quadratic equations that cannot be solved by factorising, we use a method which can solve all quadratic equations called completing the square. we use this later when studying circles in plane analytic geometry. completing the square comes from considering the special formulas that we met in square of a sum and square of a difference earlier:. Complete the square by adding \ (\left (\frac {b} {2}\right)^2\) to both sides. rewrite the quadratic side as a square by factoring. the form is always \ (\left (x \frac {b} {2}\right)^2\). square root both sides. remember the ±. solve for x. solve 0 = x2 7 x 6 by completing the square. Use this tool to practice solving quadratic equations by completing the square. instructions: enter your answers in the correct format: use ^ for exponents. example: (x 3)^2 9. use ( ) for brackets and for operations. make sure your answers are simplified. Solve quadratic equations by factorising, using formulae and completing the square. each method also provides information about the corresponding quadratic graph. some quadratics cannot be.
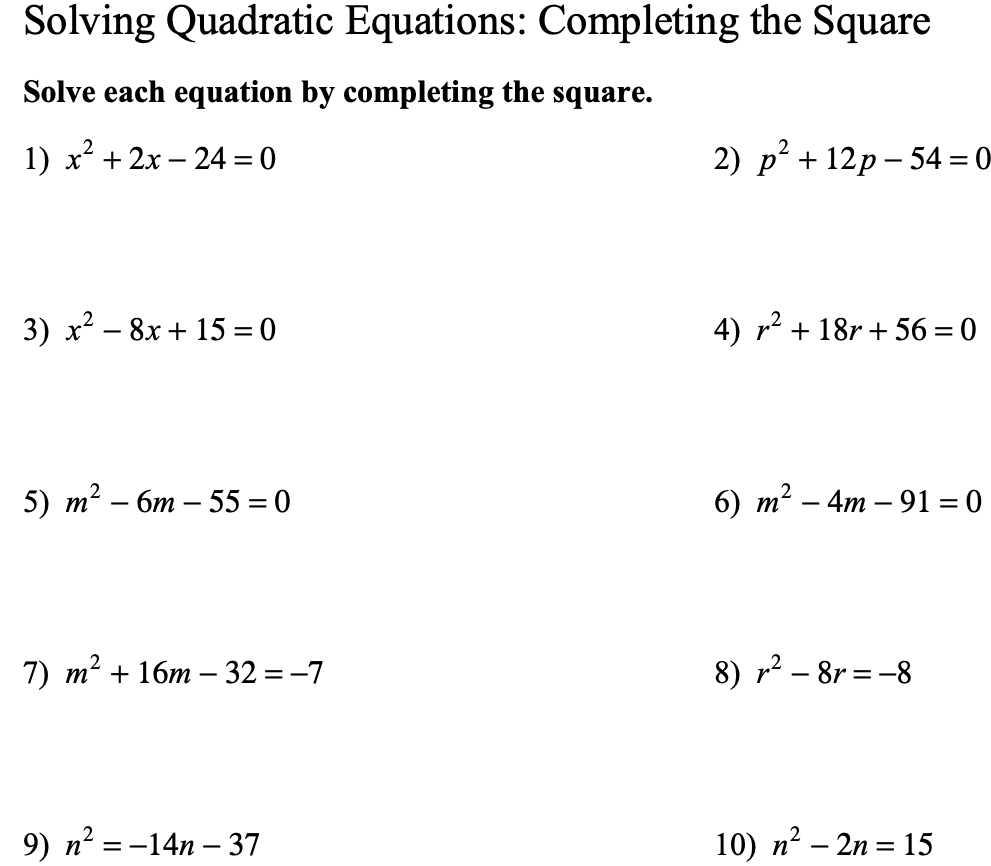
Solved Solving Quadratic Equations Completing The Square Chegg Complete the square by adding \ (\left (\frac {b} {2}\right)^2\) to both sides. rewrite the quadratic side as a square by factoring. the form is always \ (\left (x \frac {b} {2}\right)^2\). square root both sides. remember the ±. solve for x. solve 0 = x2 7 x 6 by completing the square. Use this tool to practice solving quadratic equations by completing the square. instructions: enter your answers in the correct format: use ^ for exponents. example: (x 3)^2 9. use ( ) for brackets and for operations. make sure your answers are simplified. Solve quadratic equations by factorising, using formulae and completing the square. each method also provides information about the corresponding quadratic graph. some quadratics cannot be.

Solving Quadratic Equations By Completing The Square Examples With Solve quadratic equations by factorising, using formulae and completing the square. each method also provides information about the corresponding quadratic graph. some quadratics cannot be.
