
Lecture 03 Trusses And Frames Statically Determinate Trusses A truss is called statically determinate if all the unknown forces, i.e., the forces in the members and the forces at the supports, can be determined from the equilibrium conditions. let a plane truss be composed of m members connected through j joints, and let the number of support reactions be r. Trusses are statically determinate when the entire bar forces can be determined from the equations of statics alone. otherwise the truss is statically indeterminate. a truss may be statically (externally) determinate or indeterminate with respect to the reactions (more than 3 or 6 reactions in 2d or 3d problems respectively).
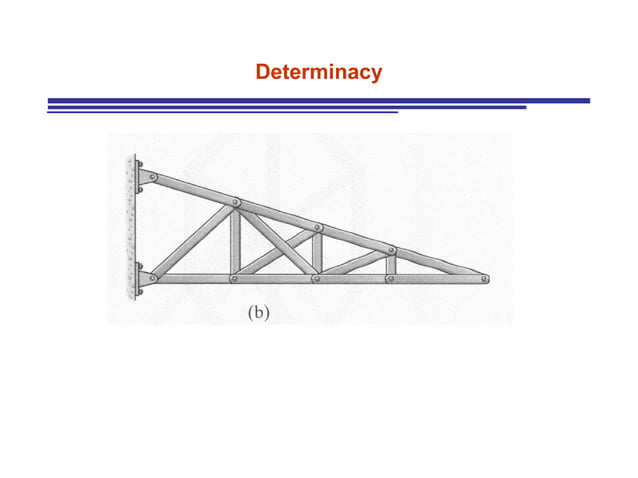
Trusses Analysis Of Statically Determinate Classify each of the trusses in the figure below as stable, unstable, statically determinate, or statically indeterminate. the trusses are subjected to arbitrary external loadings that are assumed to be known and can act anywhere on the trusses. externally stable, since the reactions are not concurrent or parallel. since b = 19,. Determinacy of trusses. test covers three categories: truss is statically determinate: m r = 2j. if m r < 2j truss is unstable. if m r > 2j truss is statically indeterminate. note. tests are necessary but not sucient. for our three test cases: test structure a: r = 3, m = 7, and j = 5. m r 2j =0! statically determinate. Classify each of the trusses as stable, unstable, statically determinate or statically indeterminate. the trusses are subjected to arbitrary external loadings that are assumed to be known & can act anywhere on the trusses. determine the force in each member of the roof truss as shown. state whether the members are in tension or compression. The document discusses statically determinate trusses, which are structures composed of slender members joined together at their endpoints. it defines two types of trusses planar trusses that lie in a single plane, and space trusses that form three dimensional structures.
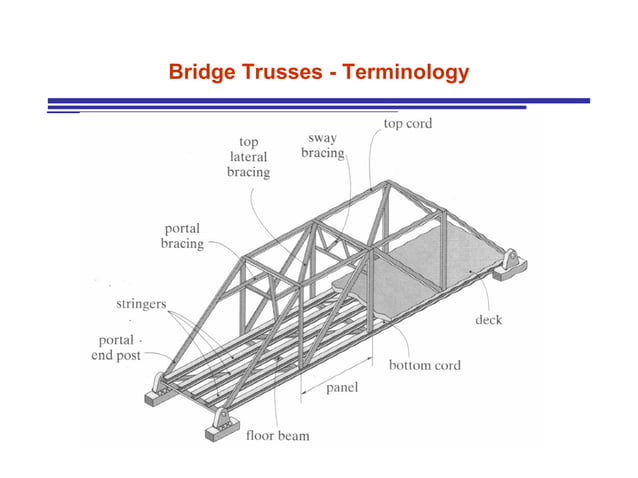
Trusses Analysis Of Statically Determinate Classify each of the trusses as stable, unstable, statically determinate or statically indeterminate. the trusses are subjected to arbitrary external loadings that are assumed to be known & can act anywhere on the trusses. determine the force in each member of the roof truss as shown. state whether the members are in tension or compression. The document discusses statically determinate trusses, which are structures composed of slender members joined together at their endpoints. it defines two types of trusses planar trusses that lie in a single plane, and space trusses that form three dimensional structures. The document analyzes statically determinate trusses and provides an example problem to determine forces in members of a roof truss structure. it also discusses methods for analyzing trusses using equilibrium equations, as well as virtual work principles for calculating truss deflections. The foregoing approach becomes particularly useful when applied to large complex structures. in engineering practice, it may be necessary to solve trusses with hundreds or even thousands of structural members. linear equations provide one powerful approach for gaining insight into the behavior of these structures. The document provides an overview of statically determinate trusses, defining them as plane structures made of slender members joined at their ends. it categorizes trusses into simple, compound, and complex types, and outlines methods for analyzing them through joint and section methods. A truss is called statically determinate if all the unknown forces, i.e., the forces in the members and the forces at the supports, can be determined from the equilibrium conditions. let a plane truss be composed of m members connected through j joints, and let the number of support reactions be r.
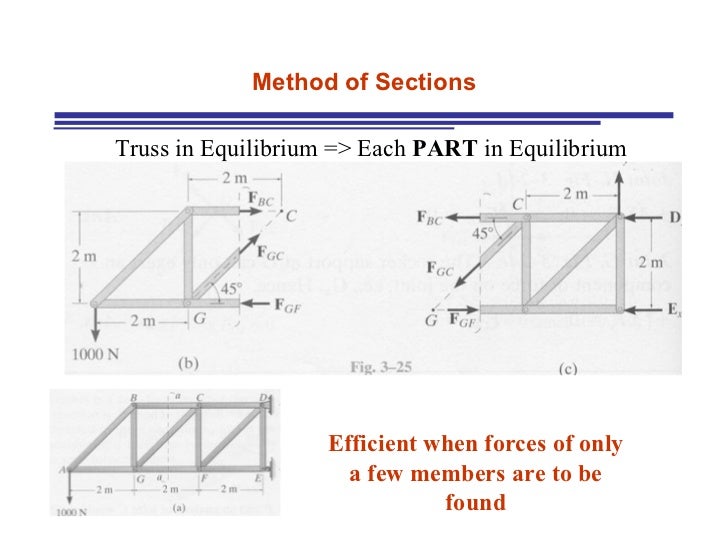
Trusses Analysis Of Statically Determinate The document analyzes statically determinate trusses and provides an example problem to determine forces in members of a roof truss structure. it also discusses methods for analyzing trusses using equilibrium equations, as well as virtual work principles for calculating truss deflections. The foregoing approach becomes particularly useful when applied to large complex structures. in engineering practice, it may be necessary to solve trusses with hundreds or even thousands of structural members. linear equations provide one powerful approach for gaining insight into the behavior of these structures. The document provides an overview of statically determinate trusses, defining them as plane structures made of slender members joined at their ends. it categorizes trusses into simple, compound, and complex types, and outlines methods for analyzing them through joint and section methods. A truss is called statically determinate if all the unknown forces, i.e., the forces in the members and the forces at the supports, can be determined from the equilibrium conditions. let a plane truss be composed of m members connected through j joints, and let the number of support reactions be r.
