
Calculus Iii Surface Integrals Of Vector Fields Pdf Understand how to responsibly use this work by visiting ‘using thinkswap resources correctly’. these are the documents you will have access to if you purchase the "surface integrals of vector fields " pack. Discuss the notions of limits, continuity, derivatives and integrals of real valued and vector valued functions of many variables. most of the time, extending these familiar notions from one to.

Surface Integrals Notes Ma Uy 2114 Studocu Mh2100 notes 12 1. surface integrals of vector fields example 1. evaluate zz f · ds s where s is the top half of the sphere x2 y 2 z 2 = 36 with upward pointing normals, and f = h0, 0, zi. solution. in spherical co ordinates, the upper hemisphere s is. This course covers calculus in 3 dimensions and thus includes content on vectors, vector fields and coordinate change. it is taught by gary greeves which is a student favourite professor as he is articulate and knowledgeable. the content covered is: the workload is generally manageable but the lecutres might seem a bit content heavy. Surface integral of scalar field given a scalar field f (x, y, z) and a surface s, our aim is to define a surface integral: zz s f (x, y, z) ds. to do this, we shall repeat the following familiar procedures: i partition the domain (surface) s ; i form riemann sums; i take limit. View notes12(4).pdf from math 2100 at nanyang technological university. mh2100 notes 12 1. surface integrals of vector fields example 1. evaluate zz f · ds s where s is the top half of the sphere x2.
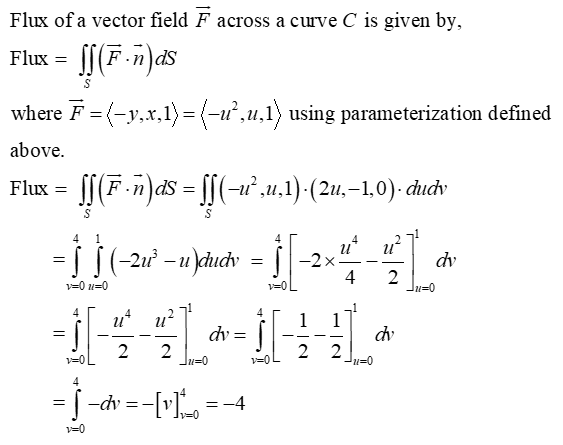
Answered Surface Integrals Of Vector Fields Find Bartleby Surface integral of scalar field given a scalar field f (x, y, z) and a surface s, our aim is to define a surface integral: zz s f (x, y, z) ds. to do this, we shall repeat the following familiar procedures: i partition the domain (surface) s ; i form riemann sums; i take limit. View notes12(4).pdf from math 2100 at nanyang technological university. mh2100 notes 12 1. surface integrals of vector fields example 1. evaluate zz f · ds s where s is the top half of the sphere x2. S f→ = yz →i x →j 3y 2 k → and s is the surface of the solid bounded by x2 y 2 = 4, z = x − 3, and z = x 2 with the negative orientation. note that all three surfaces of this solid are included in s . Search for over 200,000 study notes and past assignments! download study resources by swapping your own or buying exchange credits. study from your library anywhere, anytime. access study resources for mh2100 calculus iii. find notes, essays, projects and summaries submitted by nanyang technological university past students. sign up free. Given a vector field \(\vec f\) with unit normal vector \(\vec n\) then the surface integral of \(\vec f\) over the surface \(s\) is given by, \[\iint\limits {s}{{\vec f\centerdot d\vec s}} = \iint\limits {s}{{\vec f\centerdot \vec n\,ds}}\]. Try to do the same things as in calculus i and ii, but in higher dimensions. in this course, we shall discuss the notions of limits, continuity, derivatives and integrals of real valued and vector valued functions of many variables. most of the time, extending these familiar notions from one.
