
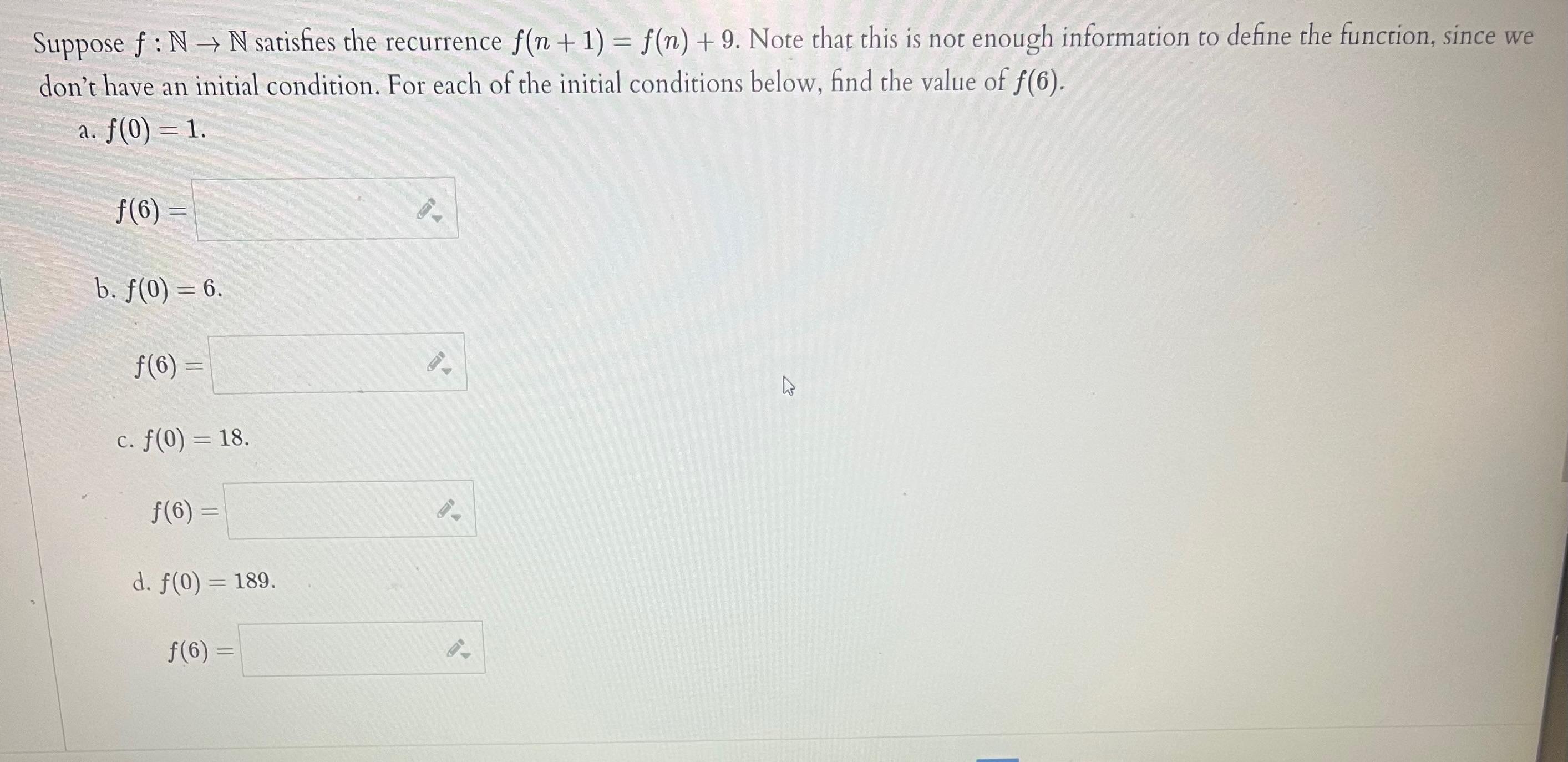
This Is A Discrete Math Question About Proofs By Chegg This is from the jay cummings proofs textbook for discrete math. your solution’s ready to go! our expert help has broken down your problem into an easy to learn solution you can count on. Proofs by contraposition ( 2 2 marks) a) consider three integers a,b and m, with m>0. we say that a is congruent to b modulo m, and we write a≡b(modm), iff m divides a−b. now, consider four integers a,b,m and n, with m>0 and n>0; show that if a≡b(modmn) then a≡b(modm). b) prove that for any real number x, if x3 is irrational then x is irrational.
Solved Discrete Math Chegg Use the pa as your study guide. try not to memorize the answers if you find yourself struggling with certain questions. take the pa multiple times if necessary. make the effort to really understand how to answer the questions on the pa correctly. consider getting yourself a subscription to chegg. Thus we can prove that whenever q is true, p → q is also true. study with quizlet and memorize flashcards containing terms like direct proof introduction, contrapositive proof introduction, contradiction proof introduction and more. There exists a this set covers topics, 1.1, 1.3, 1.4, 1.6, 1.7, and 2.1. learn with flashcards, games, and more — for free. Note: this question bank may not include some topics. final exam topics: 1. set theory (cardinality, relationships, operations, identities) 2. cartesian products 3. propositional logic & logic circuits 4. truth tables 5. logic laws (proofs) 6. translating propositions and negations 7. proofs (direct, by case, contradiction) 8.
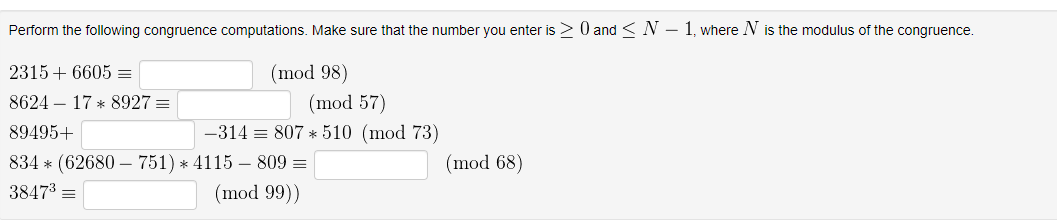
Solved Discrete Math Chegg There exists a this set covers topics, 1.1, 1.3, 1.4, 1.6, 1.7, and 2.1. learn with flashcards, games, and more — for free. Note: this question bank may not include some topics. final exam topics: 1. set theory (cardinality, relationships, operations, identities) 2. cartesian products 3. propositional logic & logic circuits 4. truth tables 5. logic laws (proofs) 6. translating propositions and negations 7. proofs (direct, by case, contradiction) 8. I have a discrete math exam coming up in 4 days and i have come to the realization i have no idea how to do proofs. our professor has never taught us any of these proof methods like direct proofs, proof by contraposition, contradiction, and induction that i have seen in trevtutor’s playlist. Submit your question to a subject matter expert. a) prove that for any integers a and b we have a2−4b =2. b) consider two positive real numbers x and y. show that (x y) 2 (the arithmetic mean of x and y ) is greater than or equal to (xy) (the geometric mean of x and y ). a) prove that for any integers a and b we have a2−4b =2. Discrete math is where a math program starts to diverge from algebra and calculus style memorization of rules towards nothing but proofs. linear algebra goes further into the realm of proofs. i'd recommend office hours, tutoring, and or a study group next time around. Quiz yourself with questions and answers for discrete math exam 1 (proofs), so you can be ready for test day. explore quizzes and practice tests created by teachers and students or create one from your course material.

Solved This Is Discrete Math Chegg I have a discrete math exam coming up in 4 days and i have come to the realization i have no idea how to do proofs. our professor has never taught us any of these proof methods like direct proofs, proof by contraposition, contradiction, and induction that i have seen in trevtutor’s playlist. Submit your question to a subject matter expert. a) prove that for any integers a and b we have a2−4b =2. b) consider two positive real numbers x and y. show that (x y) 2 (the arithmetic mean of x and y ) is greater than or equal to (xy) (the geometric mean of x and y ). a) prove that for any integers a and b we have a2−4b =2. Discrete math is where a math program starts to diverge from algebra and calculus style memorization of rules towards nothing but proofs. linear algebra goes further into the realm of proofs. i'd recommend office hours, tutoring, and or a study group next time around. Quiz yourself with questions and answers for discrete math exam 1 (proofs), so you can be ready for test day. explore quizzes and practice tests created by teachers and students or create one from your course material.

Solved I Have A Question About Discrete Math Can You Chegg Discrete math is where a math program starts to diverge from algebra and calculus style memorization of rules towards nothing but proofs. linear algebra goes further into the realm of proofs. i'd recommend office hours, tutoring, and or a study group next time around. Quiz yourself with questions and answers for discrete math exam 1 (proofs), so you can be ready for test day. explore quizzes and practice tests created by teachers and students or create one from your course material.
