
Trigonometric Function Pdf Pdf We define the cosine, sine and tangent as functions of all real numbers. these trigonometric functions are extremely important in science, engineering and mathematics, and some familiarity with them will be assumed in most first year university mathematics courses. In this chapter, we will generalise the concept of trigonometric ratios to trigonometric functions and study their properties. angle is a measure of rotation of a given ray about its initial point. the original ray is. called the initial side and the final position of the ray after rotation is called the terminal side of the angle.

Trigonometric Functions Pdf Analyze math trigonometry – contains free trigonometry tutorials and problems. uses java applets to explore important topics interactively. an important student resource for any high school or college math student is a schaum’s outline. 3.5: trigonometric functions reference evans 6.1 consider a right angled triangle with angle θ and side lengths x, y and h as shown: θ x y h the trigonometric functions sine, cosine and tangent of θ are defined as: sinθ = opposite hypotenuse = y h, cosθ = adjacent hypotenuse = x h tanθ = opposite adjacent = y x = sinθ cosθ 71. Section 7 trigonometric functions and their applications key ideas • the basic trigonometry ratios aresine, cosine, tangent, cosecant, secant and cotangent • trigonometry functions relate an angle of a right angled triangle to ratios of two side lengths. • 30°, 45° , 60° are examples of special angles. the trigonometry ratios. Trigonometric functions tutoring and learning centre, george brown college 2014 georgebrown.ca tlc this worksheet covers the basic characteristics of the sine, cosine, tangent, cotangent, secant, and cosecant trigonometric functions. sine function: f(x) = sin (x) • graph.

Chap 8 Trigonometric Functions Pdf Section 7 trigonometric functions and their applications key ideas • the basic trigonometry ratios aresine, cosine, tangent, cosecant, secant and cotangent • trigonometry functions relate an angle of a right angled triangle to ratios of two side lengths. • 30°, 45° , 60° are examples of special angles. the trigonometry ratios. Trigonometric functions tutoring and learning centre, george brown college 2014 georgebrown.ca tlc this worksheet covers the basic characteristics of the sine, cosine, tangent, cotangent, secant, and cosecant trigonometric functions. sine function: f(x) = sin (x) • graph. We look at trigonometric functions and their derivatives. consider an angle with origin (vertex) at the origin of the coordinate system and two rays where the initial side ray is along the x axis and terminal side ray is at the end of a rotation of angle θ. •specify the domain and the range of the three trigonometric functions f(x) = sinx, f(x) = cosx and f(x) = tanx, •understand the difference between each function expressed in degrees and the corresponding function expressed in radians, •express the periodicity of each function in either degrees or radians,. We use the values of the inside trig function to make a triangle, then we choose the appropriate values based on the outside function. without using a calculator, evaluate the exact value of each. Given the unit circle, x 2 y 2 = 1 , where x = cos and y = sin , we can define the three pythagorean identities below. each negative angle identity is based on the symmetry of the graph of each trigonometric function. even. f ( − x ) = f ( x ) . odd functions are symmetric about the origin, similar to the cubic function f ( x ) = x 3.

Ch5 Trigonometric Functions Pdf Trigonometric Functions We look at trigonometric functions and their derivatives. consider an angle with origin (vertex) at the origin of the coordinate system and two rays where the initial side ray is along the x axis and terminal side ray is at the end of a rotation of angle θ. •specify the domain and the range of the three trigonometric functions f(x) = sinx, f(x) = cosx and f(x) = tanx, •understand the difference between each function expressed in degrees and the corresponding function expressed in radians, •express the periodicity of each function in either degrees or radians,. We use the values of the inside trig function to make a triangle, then we choose the appropriate values based on the outside function. without using a calculator, evaluate the exact value of each. Given the unit circle, x 2 y 2 = 1 , where x = cos and y = sin , we can define the three pythagorean identities below. each negative angle identity is based on the symmetry of the graph of each trigonometric function. even. f ( − x ) = f ( x ) . odd functions are symmetric about the origin, similar to the cubic function f ( x ) = x 3.
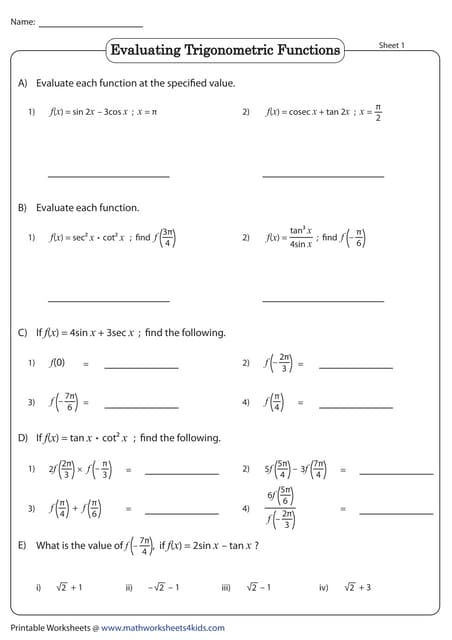
Trigonometric Functions 1 Pdf We use the values of the inside trig function to make a triangle, then we choose the appropriate values based on the outside function. without using a calculator, evaluate the exact value of each. Given the unit circle, x 2 y 2 = 1 , where x = cos and y = sin , we can define the three pythagorean identities below. each negative angle identity is based on the symmetry of the graph of each trigonometric function. even. f ( − x ) = f ( x ) . odd functions are symmetric about the origin, similar to the cubic function f ( x ) = x 3.
