
Matrices Mathematical Physics Iv Phht 411 Pdf Matrix This document provides a tutorial on matrices and determinants. it includes examples of performing operations on matrices such as addition, multiplication, and finding determinants. it also gives word problems that can be represented with matrices, such as production quantities at different plants, material costs, and revenue from multiple. We would like to develop the subject, explaining both its mathematical structure and some of its physics applications. in this section, we introduce the “arena” for linear algebra: vector spaces.

Presentation The Matrix Pdf Quadratic Equation Trigonometric Video answers for all textbook questions of chapter 4, matrix calculations , problems in theoretical physics. introductory problems volume 1 by numerade for educators. Tutorial 4 mam2084f, 2025, uct. this tutorial tackles the concepts from the third week of lectures determinants, ad joints and cramer’s rule. consider the following 4 × 4 matrix; a = 1 2 1 1. 3 2 x 1. 2 0 1 2 − 1 2 3 0 . compute the co factor of the entry x in this matrix. solution: to compute the co factor we need to compute the minor. Matrix mathematics theory, facts, and formulas dennis s. bernstein princeton university press princeton and oxford. You should be able to: add and subtract matrices that conform for addition and subtraction; multiply a matrix by a scalar; multiply matrices that conform for multiplication; translate between a system of linear equations and its coefficient matrix & augmented matrix; write systems of linear equations as a matrix equation ax = b.

Can Anyone Help In Understanding The Matrix Form Of These Two Equations Matrix mathematics theory, facts, and formulas dennis s. bernstein princeton university press princeton and oxford. You should be able to: add and subtract matrices that conform for addition and subtraction; multiply a matrix by a scalar; multiply matrices that conform for multiplication; translate between a system of linear equations and its coefficient matrix & augmented matrix; write systems of linear equations as a matrix equation ax = b. Matrix notation allows the two equations 1x 1y = b 1 1x 1y = b 2 to be expressed as 1 1 1 1 x y = b 1 b 2 or as az = b, where a = 1 1 1 1 ; z = x y ; and b = b 1 b 2 : here a;z;b are respectively: (i) thecoe cient matrix; (ii) thevector of unknowns; (iii) thevector of right hand sides. university of warwick, ec9a0 maths for economists peter j. The document discusses computing the inverse of a matrix using two methods: (1) using the formula involving the adjoint matrix and (2) using elementary row operations. it provides examples of finding the inverse of 2x2 and 3x3 matrices using each method. it also reviews the definitions and types of elementary row operations. This document contains 15 multiple choice and true false questions about matrix operations and properties. some questions ask to find sums, differences, products, and transposes of matrices. other questions test properties like whether certain operations are defined, whether matrices are equal, and conditions for matrix equality. Discover the practical applications of matrices in various physics branches from classical mechanics to advanced concepts, rounded off with a dive into theoretical matrix models. unravel the significant impact of matrices in shaping the understanding of physics. what are the basic matrix operations used in physics?.
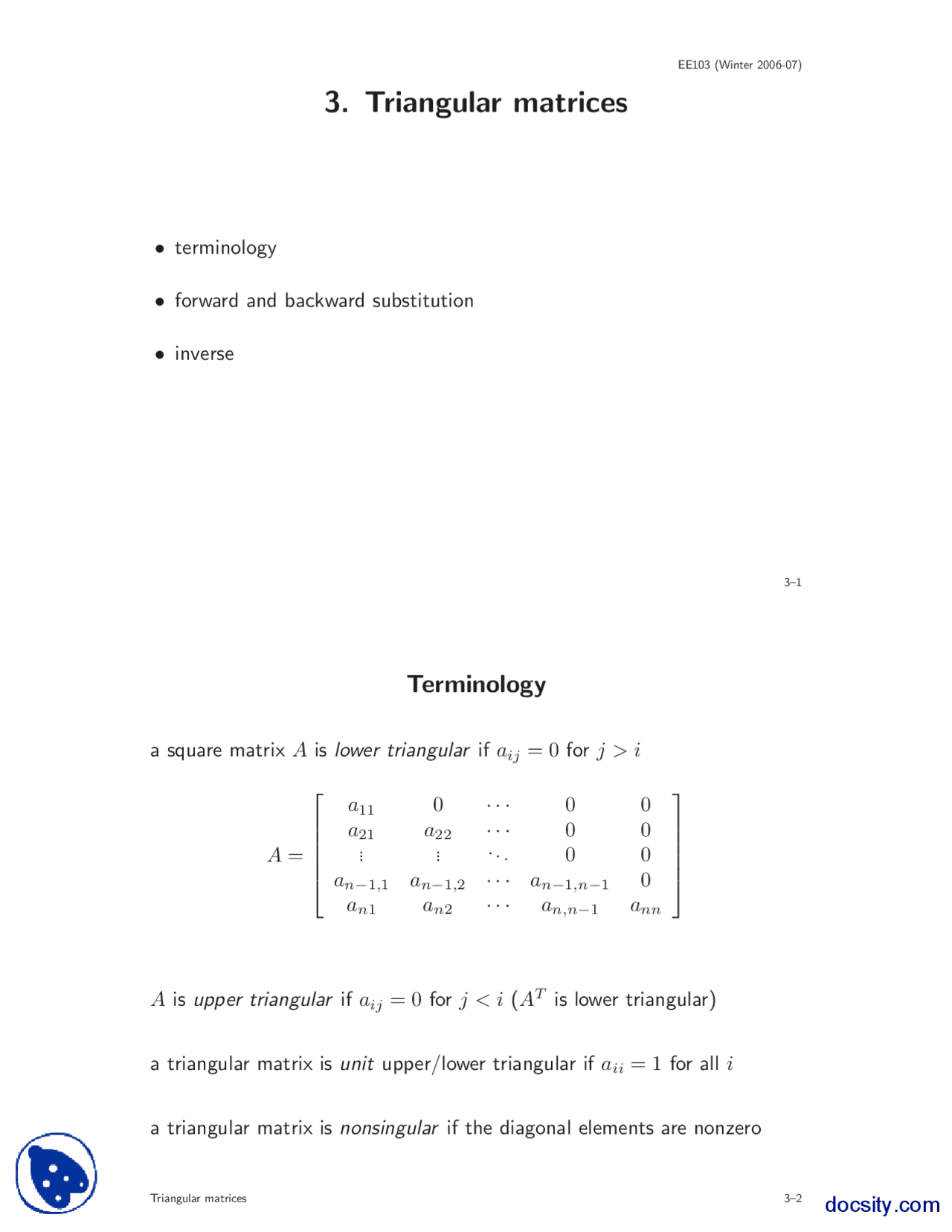
Triangular Matrices Physics Lecture Handouts Docsity Matrix notation allows the two equations 1x 1y = b 1 1x 1y = b 2 to be expressed as 1 1 1 1 x y = b 1 b 2 or as az = b, where a = 1 1 1 1 ; z = x y ; and b = b 1 b 2 : here a;z;b are respectively: (i) thecoe cient matrix; (ii) thevector of unknowns; (iii) thevector of right hand sides. university of warwick, ec9a0 maths for economists peter j. The document discusses computing the inverse of a matrix using two methods: (1) using the formula involving the adjoint matrix and (2) using elementary row operations. it provides examples of finding the inverse of 2x2 and 3x3 matrices using each method. it also reviews the definitions and types of elementary row operations. This document contains 15 multiple choice and true false questions about matrix operations and properties. some questions ask to find sums, differences, products, and transposes of matrices. other questions test properties like whether certain operations are defined, whether matrices are equal, and conditions for matrix equality. Discover the practical applications of matrices in various physics branches from classical mechanics to advanced concepts, rounded off with a dive into theoretical matrix models. unravel the significant impact of matrices in shaping the understanding of physics. what are the basic matrix operations used in physics?.

Matrix Theory Pdf This document contains 15 multiple choice and true false questions about matrix operations and properties. some questions ask to find sums, differences, products, and transposes of matrices. other questions test properties like whether certain operations are defined, whether matrices are equal, and conditions for matrix equality. Discover the practical applications of matrices in various physics branches from classical mechanics to advanced concepts, rounded off with a dive into theoretical matrix models. unravel the significant impact of matrices in shaping the understanding of physics. what are the basic matrix operations used in physics?.
