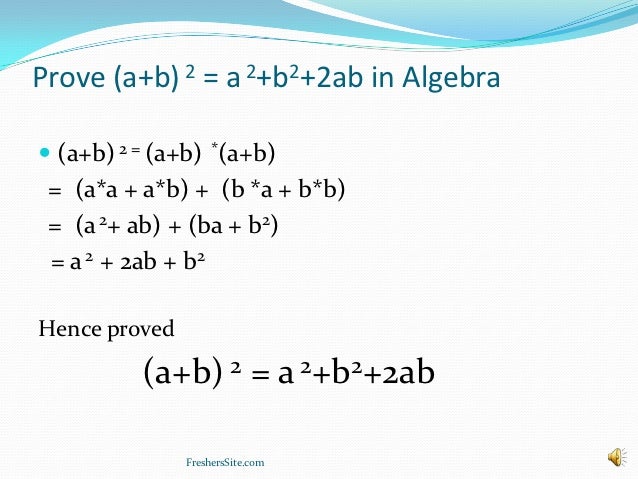
Why Is A B 2 A2 B2 2ab (a b)^2 = (a b) x (a b) = a x (a b) b x (a b) in other words, each term in the parentheses is getting multiplied by (a b). so we're not "squaring" the individual a and b, because that would mean *only* multiplying a by a, and *only* multiplying b by by. Specifically, (a b) 2 generally cannot equal (a 2 b 2), because with (a b) 2, the same thing is being multiplied everywhere (namely, (a b)), while with (a 2 b 2), a and b are being multiplied by different things (namely, a and b respectively).

Why Is A B 2 A2 B2 2ab Assume that $a$ and $b$ are both $n\times n$ matrices, how would i prove that $(a b)^2 = a^2 2ab b^2$ for any $n\times n$ matrices?. The reason is that (a b)2 (a b) 2 is nothing more than shorthand notation for (a b)(a b) (a b) (a b). foiling this expression gives. No, the formula (a b) 2 = a 2 2ab b 2 is a property of numbers called the binomial expansion, and it does not apply to matrices. in general, matrix operations such as addition and multiplication have different rules and properties than those of numbers. This document proves the formula (a b)2 = a2 b2 2ab using both geometric and algebraic approaches. geometrically, it represents a b as a line segment and draws the squares and rectangles that make up (a b)2. algebraically, it expands (a b)2 using the distributive property and collects like terms.
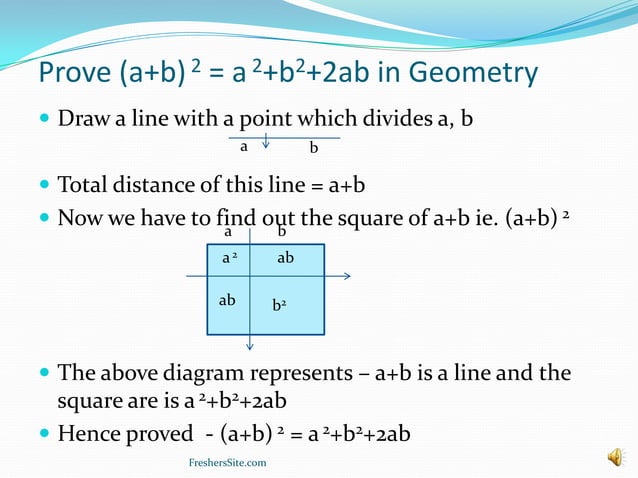
Why Is A B 2 A2 B2 2ab Ppt No, the formula (a b) 2 = a 2 2ab b 2 is a property of numbers called the binomial expansion, and it does not apply to matrices. in general, matrix operations such as addition and multiplication have different rules and properties than those of numbers. This document proves the formula (a b)2 = a2 b2 2ab using both geometric and algebraic approaches. geometrically, it represents a b as a line segment and draws the squares and rectangles that make up (a b)2. algebraically, it expands (a b)2 using the distributive property and collects like terms. For all square matrices $a$ and $b$ of the same size, it is true that $(a b)^2 = a^2 2ab b^2$? (2 answers). Explain why each of the following algebraic rules will not work in general when the real numbers a and b are replaced by n × n matrices a and b. (a) (a b)2 = a2 2ab b2 (b) (a b) (a b)=a2 b2. there are 2 steps to solve this one. We know (a b)2 = (a b) (a b) ⇒ (a b)2 = a (a b) b (a b) ∴ (a b)2 = a2 ab ba b2. for the equation, (a b)2 = a2 2ab b2 to hold, we need ab = ba that is the matrices a and b must satisfy the commutative property for multiplication. however, here it is not mentioned that ab = ba. therefore, ab ≠ ba. thus,. A2b2=(a b)2. the formula "a2 b2=(a b)2" is a cornerstone of geometry, expressing the pythagorean theorem and its applications. here are six key aspects that illuminate its significance: geometric relationship: expresses the relationship between the sides of a right triangle. algebraic identity: a true equation that holds for all values of a and b.
