
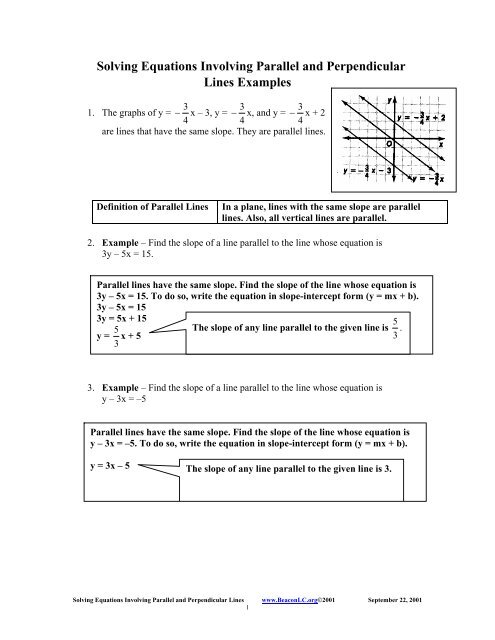
Writing Equations Of Parallel And Perpendicular Lines The calculator will find the equation of the parallel perpendicular line to the given line passing through the given point, with steps shown. for drawing lines, use the graphing calculator. We can find the equation of two lines which are parallel or perpendicular and prove whether the lines are parallel or perpendicular. parallel lines. lines which are parallel always have the sameslopeor value for m, but a differenty intercept or value for b.
Writing Equations Of Parallel And Perpendicular Lines Through A Given Equations of parallel and perpendicular lines. in this tutorial, you will learn how to construct a line that is either parallel or perpendicular to a given reference line and passing through a fixed point. to be successful in solving this kind of problem, you need to have some background knowledge about the line itself. Learn how to find equations of lines, parallel or perpendicular to a given line, passing through a given point, and see examples that walk through sample problems step by step. How to: given the equation of a function and a point through which its graph passes, write the equation of a line parallel to the given line that passes through the given point. find the slope of the function. substitute the given values into either the general point slope equation or the slope intercept equation for a line. simplify. Writing equations of parallel and perpendicular lines write the slope intercept form of the equation of the line described. 1) through: ( , ), parallel to y x 2) through: ( , ), parallel to x 3) through: ( , ), parallel to y x.

Writing Equations Of Lines Parallel And Perpendicular To A Given Line How to: given the equation of a function and a point through which its graph passes, write the equation of a line parallel to the given line that passes through the given point. find the slope of the function. substitute the given values into either the general point slope equation or the slope intercept equation for a line. simplify. Writing equations of parallel and perpendicular lines write the slope intercept form of the equation of the line described. 1) through: ( , ), parallel to y x 2) through: ( , ), parallel to x 3) through: ( , ), parallel to y x. Steps to write an equation of a line perpendicular to a given line and going through a given point: 1. make sure the equation is solved for ‘y’ 2. find the slope from the given equation and find its opposite recirpocal (that means ‘flip it’ and ‘change its sign’) ï. substitute in values for x1 1y and m into the general equation x. Make a conjecture about how you can use equations to determine whether two lines are parallel or perpendicular. write a linear function that has a different slope than the graph of f. repeat. d. parts (a) and (b) using this function. do the results support your conjecture in part (c)? e. Given the equation of a function and a point through which its graph passes, write the equation of a line perpendicular to the given line. find the slope of the function. determine the negative reciprocal of the slope. substitute the new slope and the values for and from the coordinate pair provided into ; solve for . write the equation of the. Write an equation of a line parallel to the line shown that passes through (−3,1). then write an equation of a line perpendicular to the line shown that passes through −3,1. parallel: the line shown has a slope of 3 1 or 3. using the given point and this slope, we have − = ( ) perpendicular the opposite reciprocal of 3 is −1 3.

Solved Writing Equations Of Lines Parallel And Perpendicular Chegg Steps to write an equation of a line perpendicular to a given line and going through a given point: 1. make sure the equation is solved for ‘y’ 2. find the slope from the given equation and find its opposite recirpocal (that means ‘flip it’ and ‘change its sign’) ï. substitute in values for x1 1y and m into the general equation x. Make a conjecture about how you can use equations to determine whether two lines are parallel or perpendicular. write a linear function that has a different slope than the graph of f. repeat. d. parts (a) and (b) using this function. do the results support your conjecture in part (c)? e. Given the equation of a function and a point through which its graph passes, write the equation of a line perpendicular to the given line. find the slope of the function. determine the negative reciprocal of the slope. substitute the new slope and the values for and from the coordinate pair provided into ; solve for . write the equation of the. Write an equation of a line parallel to the line shown that passes through (−3,1). then write an equation of a line perpendicular to the line shown that passes through −3,1. parallel: the line shown has a slope of 3 1 or 3. using the given point and this slope, we have − = ( ) perpendicular the opposite reciprocal of 3 is −1 3.
